-
To explain our entire universe, the search for a fundamental theory has become increasingly prominent. To date, standard model (SM) is able to describe approximately
$ 5\% $ of our universe. Hence, the rest of the universe remains unknown to us. The Standard Model describes the three generations of quarks and leptons and their interactions (excluding gravity). Recent measurements in flavor physics, along with the existence of dark energy and dark matter, suggest the possible existence of new physics (NP) beyond the Standard Model. Consequently, the search for NP has gained special attention in particle physics. New particles and their interactions with quarks and leptons are likely sources of NP. High-energy collider experiments and precise measurements of quantum effects in observables are the two primary methods used to search for new particles and interactions. The$ b $ hadron decays provide a reliable platform to probe NP beyond the SM.Recently, different experimental collaborations, such as BaBar, Belle, and LHCb, have measured and updated the lepton flavour universality (LFU) ratios
$ {R}_{{D}^{\left(*\right)}} $ associated with flavour changing charged current (FCCC)$ b\to c\bar{l}{\nu }_{l} $ transition. The reported deviations of$ {R}_{{D}^{\left(*\right)}} $ from their corresponding SM prediction are approximately$ 3.3\sigma $ [1–3]. Furthermore, another angular observable$ {P}_{5}^{{'}} $ [4] displays hints for NP beyond the SM, which is the measurement of correlations among the trajectories of the decaying particle in$ {B}_{s}\to {K}^{\left(*\right)}{\mu }^{+}{\mu }^{-} $ and decay rate$ \mathrm{\Gamma } $ in$ {B}_{s}\to \varphi {\mu }^{+}{\mu }^{-} $ . There are numerous NP models involving new particles, such as non-universal$ Z{'} $ models [5–10], leptoquark models [11–14], and models with charged Higgs [15–17] to explain these types of anomalies. Currently, these types of transitions have attracted special attention in experiments and in phenomenological studies to explore NP. These tension between theory and experiment associated with$ b $ hadron decays raise a question in our curious mind: Can a similar inconsistency be observed in charm decays induced by$ c\to (s,d)\overline{l}{\nu }_{l} $ transitions? Most of the recent experimental measurements on the pure leptonic and semileptonic$ D $ decays, except$ {D}_{\left(s\right)}^{+}\to {\eta }^{\left({'}\right)}\overline{l}{\nu }_{l} $ decays, agree with the SM predictions. In the last few years, a significant number of theoretical efforts have been performed for searching NP contribution in$ D $ decays [18–23], and charm decays provide a special opportunity to investigate flavour physics beyond the SM in the up-sector. In Table 1, it can be observed that recent experimental results [24, 25] of branching fractions for decays$ {D}_{\left(s\right)}^{+}\to {\eta }^{\left({'}\right)}\overline{l}{\nu }_{l} $ depict tension with their corresponding SM predictions. Hence, these decays provide opportunities to explore NP. Based on these results,$ {D}_{\left(s\right)}^{+}\to {\eta }^{\left({'}\right)}\overline{l}{\nu }_{l} $ decays are examined in$ {W}^{{'}} $ model [14, 26, 27] and scalar leptoquark (LQ) model [14] to explore NP effects. For theoretical study, the heavy-to-light form factors are key input parameters. Given the nonperturbative nature, they can be parameterized using different nonperturbative methods such as quark models, QCD sum rules, lattice QCD (LQCD) [28–29], and light-cone sum rules (LCSR) [30]. Using LQCD approach [31], the SM predictions of branching fraction for$ D\to \pi \left(K\right)l{\nu }_{l} $ decays are found to be in good consistency with the experimental results [24] with large uncertainties arising from the CKM matrix elements. The LQCD approach is based on the first principles, and it is more useful to obtain a precise result for$ D\to P $ (pseudoscalar meson) transitions. The LCSR method utilizes the operator product expansion close to the light-cone, encapsulating all non-perturbative dynamics within the light-cone distribution amplitudes [32]. Furthermore, this approach is suitable to parameterize heavy-to-light transition form factors. The parametric space of NP parameters have been constrained by taking precise prediction of$ D $ mesons decays using LQCD and LCSR approaches. In the NP models, the$ W{'} $ boson and leptoquark are hypothetical beyond SM particles that couple a quark directly to a lepton unlike any particle within the SM. They both are relics of grand unified theories, which were designed to integrate various interactions in the SM at high energies. In this study, we predicted new coupling parameters using recent experimental results of branching fractions provided in Tables 4 and 5. Using these new coupling parameters, branching fraction, and forward-backward asymmetry, lepton polarization asymmetry of$ {D}_{\left(s\right)}^{+}\to {\eta }^{\left({'}\right)}\overline{l}{\nu }_{l} $ decays have been investigated to explore NP effects.Decay SM result [18] Experiment [24] $Pul{l}_{\rm SM}$ 

$ \mathcal{B}({D}_{s}^{+}\to \eta {\mu }^{+}{\nu }_{\mu })\times {10}^{-2} $ 

$ 1.52\pm 0.31 $ 

$ 2.4\pm 0.5 $ 

$ 1.5\sigma $ 

$ 2.215\pm 0.051\pm 0.052 $ [25]

$ 2.2\sigma $ 

$ {\mathcal{B}(D}_{s}^{+}\to {\eta }{{'}}{\mu }^{+}{\nu }_{\mu }) \times {10}^{-3} $ 

$ 5.64\pm 1.10 $ 

$ 11.0\pm 5.0 $ 

$ 1.05\sigma $ 

$ 8.01\pm 0.55\pm 0.31 $ [25]

$ 1.9\sigma $ 

$ {\mathcal{B}(D}_{s}^{+}\to \eta {e}^{+}{\nu }_{e})\times {10}^{-2} $ 

$ 1.55\pm 0.33 $ 

$ 2.29\pm 0.19 $ 

$ 2\sigma $ 

$ {\mathcal{B}(D}_{s}^{+}\to {\eta }{{'}}{e}^{+}{\nu }_{e})\times {10}^{-3} $ 

$ 5.91\pm 1.26 $ 

$ 7.4\pm 1.4 $ 

$ 0.8\sigma $ 

$ {\mathcal{B}(D}^{+}\to \eta {\mu }^{+}{\nu }_{\mu })\times {10}^{-3} $ 

$ 0.75\pm 0.15 $ 

$ 1.04\pm 0.11 $ 

$ 1.6\sigma $ 

$ {\mathcal{B}(D}^{+}\to {\eta }{{'}}{\mu }^{+}{\nu }_{\mu })\times {10}^{-4} $ 

$ 1.06\pm 0.20 $ 

− − $ {\mathcal{B}(D}^{+}\to \eta {e}^{+}{\nu }_{e})\times {10}^{-3} $ 

$ 0.76\pm 0.16 $ 

$ 1.11\pm 0.07 $ 

$ 2\sigma $ 

$ {\mathcal{B}(D}^{+}\to {\eta }{{'}}{e}^{+}{\nu }_{e})\times {10}^{-4} $ 

$ 1.12\pm 0.24 $ 

$ 2.0\pm 0.4 $ 

$ 1.9\sigma $ 

Table 1. Theoretical and experimental results of branching fractions and their corresponding deviation for
$ {D}_{\left(s\right)}^{+}\to {\eta }^{\left({'}\right)}\overline{l}{\nu }_{l} $ decays.The layout of this paper is as follows: In Sec. II, we provide an overview of the theoretical formulation for the effective field theory approach to examine
$ {D}_{\left(s\right)}^{+}\to {\eta }^{\left({'}\right)}\overline{\nu }_{l} $ decays. The formulation of$ {W}' $ model and scalar LQ model are presented in Sec. III. In Sec. IV, our numerical analysis is presented and a comparative study between our NP models is also conducted. We concluded our findings in Sec. V. -
Considering all possible Lorentz structures and assuming only left-handed neutrinos, the general effective Lagrangian for the
$ c\to (s,d)\overline{l}{\nu }_{l} $ transitions can be expressed as [18, 19]:$ \begin{aligned}[b]{\mathcal{L}}_{eff}=\;&-\frac{4{G}_{F}}{\sqrt{2}}{V}_{cq}^{*}\Big[\left(1+{C}_{{V}_{L}}^{l}\right){O}_{{V}_{L}}^{l}+{C}_{{V}_{R}}^{l}{O}_{{V}_{R}}^{l}+{C}_{{S}_{L}}^{l}{O}_{{S}_{L}}^{l}\\&+{C}_{{S}_{R}}^{l}{O}_{{S}_{R}}^{l}+{C}_{T}^{l}{O}_{T}^{l}\Big]+{\rm h.c} ,\end{aligned} $

(1) where
$ {G}_{F} $ denotes the Fermi constant and$ {V}_{cq} $ denotes the CKM matrix element. The four-fermion operators are defined as:$\begin{aligned}[b]{O}_{{V}_{L}}^{l}=\;&\left(\overline{q}{\gamma }^{\mu }{P}_{L}c\right)\left({\overline{\nu }}_{l}{\gamma }_{\mu }{P}_{L}l\right) ,\\ {O}_{{V}_{R}}^{l}=\;&\left(\overline{q}{\gamma }^{\mu }{P}_{R}c\right)\left({\overline{\nu }}_{l}{\gamma }_{\mu }{P}_{L}l\right) ,\\ {O}_{{S}_{L}}^{l}=\;&\left(\overline{q}{P}_{L}c\right)\left({\overline{\nu }}_{l}{P}_{R}l\right) ,\\ {O}_{{S}_{R}}^{l}=\;&\left(\overline{q}{P}_{R}c\right)\left({\overline{\nu }}_{l}{P}_{R}l\right) ,\\ {O}_{T}^{l}=\;&\left(\overline{q}{\sigma }^{\mu \nu }{P}_{L}c\right)\left({\overline{\nu }}_{l}{\sigma }_{\mu \nu }{P}_{R}l\right) , \end{aligned}$

(2) where
$ {P}_{L\left(R\right)}=(1\mp {\gamma }^{5})/2 $ and$ {C}_{i}^{l} $ ($ i={V}_{L} $ ,$ {V}_{R} $ ,$ {S}_{L} $ ,$ {S}_{R} $ and$ T $ ) are the corresponding Wilson coefficients at scale$ \mu ={m}_{c} $ , with$ {C}_{i}^{l}=0 $ in SM.Specifically, the hadronic transition is quantified using heavy-to-light form factors. These form factors, though rooted in nonperturbative dynamics, exhibit universal nature. Specifically, the hadronic matrix elements characterizing transitions from a
$ D $ meson to a pseudoscalar meson denoted as$ P(P=\eta ,\eta {'}) $ can be expressed as:$ \begin{aligned}[b]&<P\left({p}_{2}\right)|\overline{q}{\gamma }^{\mu }c|D\left({p}_{1}\right)>\\=\;&{f}_+\left({q}^{2}\right)\Bigg[{\left({p}_{1}+{p}_{2}\right)}^{\mu }-\frac{{m}_{D}^{2}-{m}_{P}^{2}}{{q}^{2}}{q}^{\mu }\Bigg]+{f}_{0}\left({q}^{2}\right)\frac{{m}_{D}^{2}-{m}_{P}^{2}}{{q}^{2}}{q}^{\mu } , \end{aligned}$

(3) $\begin{aligned}[b] <P\left({p}_{2}\right)|\overline{q}c|D\left({p}_{1}\right)>=\;&\frac{{q}_{\mu }}{{m}_{c}-{m}_{q}}<P\left({p}_{2}\right)|\overline{q}{\gamma }^{\mu }c|D\left({p}_{1}\right)>\\=\;&\frac{{m}_{D}^{2}-{m}_{p}^{2}}{{m}_{c}-{m}_{q}}{f}_{0}\left({q}^{2}\right) ,\end{aligned} $

(4) $ <P\left({p}_{2}\right)|\overline{q}{\sigma }^{\mu \nu }c|D\left({p}_{1}\right)>=-i\left({p}_{1}^{\mu }{p}_{2}^{\nu }-{p}_{1}^{\nu }{p}_{2}^{\mu }\right)\frac{2{f}_{T}\left({q}^{2}\right)}{{m}_{D}+{m}_{P}} , $

(5) where
$ {q}^{\mu } $ denotes the momentum difference$ {\left({p}_{1}-{p}_{2}\right)}^{\mu } $ , while$ {f}_{+}\left({q}^{2}\right) $ and$ {f}_{0}\left({q}^{2}\right) $ correspond to a pair of QCD form factors that encapsulate the dynamics of strong interactions. These form factors satisfy the condition$ {f}_{+}\left(0\right)={f}_{0}\left(0\right) $ , signifying a shared value at zero momentum transfer. At the present moment, the LQCD form factors connected to the aforementioned transitions remain inaccessible. In this study, we used the form factors obtained from light cone sum rules (LCSR) [30], which are parameterized as:$ {F}^{i}\left({q}^{2}\right)=\frac{{F}^{i}\left(0\right)}{1-a\dfrac{{q}^{2}}{{m}_{D}^{2}}+b{\left(\dfrac{{q}^{2}}{{m}_{D}^{2}}\right)}^{2}} , $

(6) where
$ {F}^{i}\left({q}^{2}\right) $ has the flexibility to represent either of the form factors$ {f}_{+} $ or$ {f}_{0} $ . Along with the CKM matrix element, the form factors describing the dynamic of strong interaction are important for the calculation of decay rate. Mesons$ \eta $ and$ {\eta }{{'}} $ can be distinguished due to the significant involvement of the spectator quark in shaping the final state, unlike in the case of final-state hadrons$ K $ and$ \pi $ . Furthermore,$ \eta $ and$ {\eta }{{'}} $ mesons are the octet-singlet mixing state of$ \eta -{\eta }{{'}} $ gluon [33, 34]. This mixing parameter proves a deeper understanding of non-perturbative QCD confinement. The octet-singlet mixing angle$ \theta $ is within the range −10° to −23° [35]. The mixtures of the flavour$S U\left(3\right)$ octet$ {\eta }_{8} $ and singlet$ {\eta }_{0} $ can be represented by$ \left(\begin{array}{c}\eta \\ {\eta }{{'}}\end{array}\right)=\left(\begin{array}{cc}\mathrm{cos}\theta & -\mathrm{sin}\theta \\ \mathrm{sin}\theta & \mathrm{cos}\theta \end{array}\right)\left(\begin{array}{c}{\eta }_{8}\\ {\eta }_{0}\end{array}\right) , $

(7) where
$ {\eta }_{8}=(u\overline{u}+d\overline{d}-2s\overline{s})/\sqrt{6} $ and$ {\eta }_{0}=(u\overline{u}+d\overline{d}+s\overline{s})/\sqrt{3} $ . For the quark-flavour basis$ {\eta }_{q}=(u\overline{u}+d\overline{d})/\sqrt{2} $ and$ {\eta }_{s}=s\overline{s} $ can be expressed as [36]:$ \left(\begin{array}{c}\eta \\ {\eta }{{'}}\end{array}\right)=\left(\begin{array}{cc}\mathrm{cos}\varphi & -\mathrm{sin}\varphi \\ \mathrm{sin}\varphi & \mathrm{cos}\varphi \end{array}\right)\left(\begin{array}{c}{\eta }_{q}\\ {\eta }_{s}\end{array}\right) , $

(8) where
$ \varphi $ denotes the mixing angle between the state of$ {\eta }_{q} $ and$ {\eta }_{s} $ . Due to the indefinite gluon content in$ {\eta }^{\left({'}\right)} $ , large uncertainty may arise. Hence, the estimation of hadronic form factor is very important for the theoretical calculations. Using LQCD [37] and LCSR [38, 39],$ {f}_{+}^{{\eta }^{\left({'}\right)}}\left(0\right) $ are estimated by considering the mixture of quarks and gluons for$ \eta $ and$ {\eta }{{'}} $ mesons. In the quark flavour basis, the$ \eta -{\eta }{{'}} $ mixing angle is connected to the branching ratios of$ D $ and$ {D}_{s} $ by the relation${\mathrm{cot}}^{4}\varphi = \dfrac{\mathrm{\Gamma }({D}_{s}^{+}\to {\eta }{{'}}{e}^{+}{\nu }_{e})/\mathrm{\Gamma }({D}_{s}^{+}\to \eta {e}^{+}{\nu }_{e})}{\mathrm{\Gamma }({D}^{+}\to {\eta }{{'}}{e}^{+}{\nu }_{e})/\mathrm{\Gamma }({D}^{+}\to \eta {e}^{+}{\nu }_{e})}$ , where a possible gluon component is canceled [40]. A supplementary constraint on the gluonium function in$ {\eta }{\left({'}\right)} $ mesons is provided by the determination of$ \varphi $ , enhancing the knowledge of nonperturbative QCD dynamics and aiding theoretical study of$ D $ meson decays involving$ {\eta }{\left({'}\right)} $ mesons. The KLOE collaboration [41] predicted the mixing angle$ \varphi ={\left(39.7\pm 0.7\right)} $ ° and$(41.5\pm {0.3}_{\rm stat}\pm {0.7}_{\rm syst}\pm {0.6}_{\rm th})$ ° with and without gluonium content for$ {\eta }{{'}} $ respectively. Recently, BESIII Collaboration [42] has also established$\varphi =(40.1\pm {2.1}_{\rm stat}\pm {0.7}_{\rm syst})$ ° from the measurement of dynamics of$ {D}_{s}^{+}\to {\eta }^{\left({'}\right)}{e}^{+}{\nu }_{e} $ decays.The explanation of the kinematics involved in
$ D\to P\overline{l}{\nu }_{l} $ decay processes can be obtained through the helicity amplitudes. Within the framework of SM, the process where$ c $ quark transitions to$q (q = s, d)$ quark accompanied by the emission of an$ {l}^{+}{\nu }_{l} $ pair can be visualized as$ c $ quark transforming into an off-shell$ {W}^{*+} $ boson. Subsequently, this off-shell$ {W}^{*+} $ particle decays into an$ {l}^{+}{\nu }_{l} $ pair. From Eq. (1), the amplitude can be defined as [43–45]:$\mathcal{M}\left(D\to P\overline{l}{\nu }_{l}\right)=\frac{{G}_{F}}{\sqrt{2}}{V}_{cq}^{*}\sum _{k}{C}_{k}\left(\mu \right)<P|\overline{q}{\mathrm{\Gamma }}^{k}c|D>{\overline{u}}_{l}{\mathrm{\Gamma }}_{k}{v}_{\nu } , $

(9) where
$ {C}_{k}\left(\mu \right) $ denotes the Wilson coefficient with values:$ {C}_{k}\left(\mu \right)=\left\{\begin{array}{*{20}{l}}1 &{\rm for\; SM},\\ {C}_{{V}_{L,R}},{C}_{{S}_{L,R}},{C}_{T}& {\rm for\; NP},\end{array}\right. $

where
$ {\mathrm{\Gamma }}^{k} $ denotes the product of gamma matrices i.e.,$ {\mathrm{\Gamma }}^{k}={\gamma }^{\mu }(1\pm {\gamma }_{5}) $ and$ (1\pm {\gamma }_{5}) $ . The square of the matrix element can be expressed as the product of leptonic ($ {L}_{\mu v} $ ) and hadronic ($ {H}^{\mu v} $ ) tensors:$ {\left|\mathcal{M}\left(D\to P\stackrel-{l}{\nu }_{l}\right)\right|}^{2}=\frac{{G}_{F}^{2}}{2}{\left|{V}_{cq}\right|}^{2}\sum _{i,j}{C}_{ij}\left(\mu \right)\left({L}_{\mu v}^{ij}{H}^{\mu v,ij}\right) . $

(10) Here, superscripts
$ i,j $ denote the combination of four operators ($ V\mp A $ ), ($ S\mp P $ ) in the effective lagrangian and the product of Wilson coefficients$ {C}_{i} $ and$ {C}_{j} $ is represented by$ {C}_{ij}\left(\mu \right) $ . In the following discussion, these superscripts have been omitted for convenience. The polarization vector of the off-shell particle$ {W}^{*}\left({\epsilon}^{\mu }\right(m\left)\right) $ satisfies the orthonormality and completeness relations:$ {\epsilon}^{*\mu }\left(m\right){\epsilon}_{\mu }\left(n\right)={g}_{mn} ,\quad \sum _{m,n}{\epsilon}^{*\mu }\left(m\right){\epsilon}^{\nu }\left(n\right){g}_{mn}={g}^{\mu \nu } , $

(11) where
$ {g}_{mn}={\rm diag} \left(+,-,-,-\right) $ and$ m,n=\pm , 0,t $ represent the transverse, longitudinal and time-like polarization components. Using the aforementioned completeness relation, the product of$ {L}_{\mu v} $ and$ {H}^{\mu v} $ can be expressed as:$ {L}_{\mu v}{H}^{\mu v}=\sum _{m,{m}{{'}},n,{n}{{'}}}L\left(m,n\right)H\left({m}{{'}},{n}{{'}}\right){g}_{m{m}{{'}}}{g}_{n{n}{{'}}} , $

(12) where
$L\left(m,n\right)={L}^{\mu v}{\epsilon}_{\mu }\left(m\right) {\epsilon}_{\nu }^{*}\left(n\right)$ and$H\left(m,n\right)={H}^{\mu v} {\epsilon}_{\mu }^{*}\left(m\right){\epsilon}_{v}\left(n\right)$ denote the Lorentz invariant parameters and it is clear that their values are independent with respect to reference frame. In this context,$ H\left(m,n\right) $ and$ L\left(m,n\right) $ will be calculated in the$ D $ meson rest frame and$ l-{\nu }_{l} $ center-of-mass frame, respectively.The off-shell
$ {W}^{*+} $ encompasses four helicity states, characterized by$ {\lambda }_{W}=\pm 1 $ ,$ 0 $ (with$ J=1 $ ) and$ {\lambda }_{W}=0 $ (with$ J=0 $ ). It is important to note that only$ {W}^{*+} $ possesses a timelike polarization, and the angular momenta are denoted by$J=1\;{\rm or}\;0$ in the rest frame of$ {W}^{*} $ boson. To distinguish the two cases of$ {\lambda }_{W}=0 $ , we assigned$ {\lambda }_{W}=0 $ for$ J=1 $ and$ {\lambda }_{W}=t $ for$ J=0 $ . In the rest frame of the$ D $ meson, the direction of motion of the$ {W}^{*+} $ has been aligned along the$ Z $ - axis. In this context, the polarization vectors of the$ {W}^{*+} $ are expressed as follows$ \begin{aligned}[b]& {\epsilon}^{\mu }\left(\pm \right)=\frac{1}{2}(0, 1, \pm {\rm i}, 0) ,\\ &{\epsilon}^{\mu }\left(0\right)=-\frac{1}{\sqrt{{q}^{2}}}({q}_{3}, 0, 0,{q}_{0}) ,\\ &{\epsilon}^{\mu }\left(t\right)=-\frac{{q}^{\mu }}{\sqrt{{q}^{2}}} ,\end{aligned}$

(13) where
$ {q}^{\mu } $ denotes the four-momentum of$ {W}^{*+} $ . The polarization vectors of the virtual$ {W}^{*+} $ satisfy the aforementioned orthogonality and completeness relations.Specifically, the total matrix can be separated into two distinct components: the lepton portion and hadron portion. Interestingly, neither of these components are inherently Lorentz invariant. However, upon introducing the completeness relations of the virtual
$ {W}^{*+} $ particle, the hadron and lepton components transform into Lorentz invariant forms. This transformation allows us to adopt a specific coordinate system without compromising the integrity of the analysis. Consequently, we examine the hadron aspect in the initial state, particularly within the rest frame of$ D $ meson, while simultaneously evaluating the lepton component in the rest frame of the virtual$ {W}^{*+} $ . The helicity amplitudes of$ D\to P{W}^{*+} $ transitions are:$ {H}_{{\lambda }_{W}}^{PV}\left({q}^{2}\right)={\epsilon}_{\mu }^{*}\left({\lambda }_{W}\right)<P\left({p}_{2}\right)|\overline{q}{\gamma }^{\mu }c|D\left({p}_{1}\right)> , $

(14) $ {H}^{PS}\left({q}^{2}\right)=<P\left({p}_{2}\right)|\overline{q}c|D\left({p}_{1}\right)> , $

(15) $ {H}_{{\lambda }_{W},{\lambda }_{W}^{{'}}}^{PT}\left({q}^{2}\right)={\epsilon}_{\mu }^{*}\left({\lambda }_{W}\right){\epsilon}_{\nu }^{*}\left({\lambda }_{W}^{{'}}\right)<P\left({p}_{2}\right)|\overline{q}{\sigma }^{\mu \nu }c|D\left({p}_{1}\right)> . $

(16) Specifically,
$ D\to P{l}^{+}{\nu }_{l} $ decays have contributions from only five helicity amplitudes, which can be expressed as:$ {H}_{0}^{PV}=\frac{{f}_+\sqrt{{Q}_+{Q}_-}}{\sqrt{{q}^{2}}} ,\;\; {H}_{t}^{PV}=\frac{{f}_{0}{M}_+{M}_-}{\sqrt{{q}^{2}}} , \;\;{H}^{PS}=\frac{{f}_{0}{M}_+{M}_-}{{m}_{c}-{m}_{q}} , $

$ {H}_{0,t}^{PT}=-\frac{{f}_{T}\sqrt{{Q}_+{Q}_-}}{{M}_+} ,\;\; {H}_{1,-1}^{PT}=-\frac{{f}_{T}\sqrt{{Q}_+{Q}_-}}{{M}_+} , $

(17) where
$ {M}_{\pm }={m}_{D}\pm {m}_{P} $ and$ {Q}_{\pm }={M}_{\pm }^{2}-{q}^{2} $ . The leptonic amplitudes can be directly calculated, and the explicit results are provided in Ref. [46].Using the hadronic helicity amplitudes and leptonic amplitudes, the two-fold differential angular decay distribution of
$ D\to P{l}^{+}{\nu }_{l} $ decay can be expressed as:$\begin{aligned}[b]& \frac{{\rm d}^{2}\mathrm{\Gamma }\left(D\to P{l}^+{\nu }_{l}\right)}{{\rm d} {q}^{2}{\rm d} \cos{\theta }_{l}}\\=\;&\frac{{G}_{F}^{2}{\left|{V}_{cq}\right|}^{2}\sqrt{{Q}_+{Q}_-}}{256{\pi }^{3}{m}_{D}^{3}}{\left(1-\frac{{m}_{l}^{2}}{{q}^{2}}\right)}^{2}\\&\times\left[{q}^{2}{A}_{1}^{P}+\sqrt{{q}^{2}}{m}_{l}{A}_{2}^{P}+{m}_{l}^{2}{A}_{3}^{P}\right] ,\end{aligned} $

(18) with
$ \begin{aligned}[b]{A}_{1}^{P}=\;&{\left|{C}_{{S}_{L}}+{C}_{{S}_{R}}\right|}^{2}{\left|{H}^{PS}\right|}^{2}+{\rm Re} \left[\left({C}_{{S}_{L}}+{C}_{{S}_{R}}\right){C}_{T}^{*}\right]{H}^{PS}\left({H}_{0,t}^{PT}+{H}_{0,-1}^{PT}\right)\cos{\theta }_{l}\\&+4{\left|{C}_{T}\right|}^{2}{\left|{H}_{0,t}^{PT}+{H}_{1,-1}^{PT}\right|}^{2}{\mathrm{cos}}^{2}{\theta }_{l}+{\left|1+{{C}_{V}}_{L}+{{C}_{V}}_{R}\right|}^{2}{\left|{H}_{0}^{PV}\right|}^{2}{\mathrm{sin}}^{2}{\theta }_{l} ,\end{aligned} $

(19) $ \begin{aligned}[b]{A}_{2}^{P}=\;&2\left\{{\rm Re}\left[\left({{C}_{S}}_{L}+{{C}_{S}}_{R}\right){\left(1+{{C}_{V}}_{L}+{{C}_{V}}_{R}\right)}^{*}\right]{H}^{PS}{H}_{t}^{PV}-2{\rm Re}\left[{C}_{T}{\left(1+{{C}_{V}}_{L}+{{C}_{V}}_{R}\right)}^{*}\right]{H}_{0}^{PV}\left({H}_{0,t}^{PT}+{H}_{1,-1}^{PT}\right)\right\}\\&-2\left\{{\rm Re}\left[\left({C}_{{S}_{L}}+{{C}_{S}}_{R}\right){\left(1+{{C}_{V}}_{L}+{{C}_{V}}_{R}\right)}^{*}\right]{H}^{PS}{H}_{0}^{PV}-2{\rm Re}\left[{C}_{T}{\left(1+{{C}_{V}}_{L}+{{C}_{V}}_{R}\right)}^{*}\right]{H}_{t}^{PV}\left({H}_{0,t}^{PT}+{H}_{1,-1}^{PT}\right)\right\}\cos{\theta }_{l} ,\end{aligned} $

(20) $ {A}_{3}^{P}=4{\left|{C}_{T}\right|}^{2}{\left|{H}_{0,t}^{PT}+{H}_{1,-1}^{PT}\right|}^{2}{\mathrm{sin}}^{2}{\theta }_{l}+{\left|1+{C}_{{V}_{L}}+{C}_{{V}_{R}}\right|}^{2}({\left|{H}_{0}^{PV}\right|}^{2}{\mathrm{cos}}^{2}{\theta }_{l}-2{H}_{0}^{PV}{H}_{T}^{PV}\cos{\theta }_{l}+{\left|{H}_{t}^{PV}\right|}^{2}) , $

(21) where
$ {\theta }_{l} $ denotes the angle between the charged lepton and opposite direction of the motion of the final meson in the virtual$ {W}^{*+} $ rest frame. The forward-backward asymmetry can be defined as:$ {A}_{FB}\left({q}^{2}\right)=\dfrac{{\int }_{0}^{1}{\rm d}\cos{\theta }_{l}\dfrac{{\rm d}^{2}\mathrm{\Gamma }}{{\rm d}{q}^{2}{\rm d}\cos{\theta }_{l}}-{\int }_{-1}^{0}{\rm d}\cos{\theta }_{l}\dfrac{{\rm d}^{2}\mathrm{\Gamma }}{{\rm d}{q}^{2}{\rm d}\cos{\theta }_{l}}}{{\int }_{0}^{1}{\rm d}\cos{\theta }_{l}\dfrac{{\rm d}^{2}\mathrm{\Gamma }}{{\rm d}{q}^{2}{\rm d}\cos{\theta }_{l}}+{\int }_{-1}^{0}{\rm d}\cos{\theta }_{l}\dfrac{{\rm d}^{2}\mathrm{\Gamma }}{{\rm d}{q}^{2}{\rm d}\cos{\theta }_{l}}} , $

(22) Furthermore, the lepton polarization asymmetry can be expressed as:
$ {P}_{F}^{l}\left({q}^{2}\right)=\frac{\dfrac{{\rm d}\mathrm{\Gamma }\left({\lambda }_{l}=\dfrac{1}{2}\right)}{{\rm d}{q}^{2}}-\dfrac{{\rm d}\mathrm{\Gamma }\left({\lambda }_{l}=-\dfrac{1}{2}\right)}{{\rm d}{q}^{2}}}{\dfrac{{\rm d}\mathrm{\Gamma }\left({\lambda }_{l}=\dfrac{1}{2}\right)}{{\rm d}{q}^{2}}+\dfrac{{\rm d}\mathrm{\Gamma }\left({\lambda }_{l}=-\dfrac{1}{2}\right)}{{\rm d}{q}^{2}}} . $

(23) -
Motivated by various extended theoretical approaches of the SM for the prediction of hypothetical particles, recently, ATLAS and CMS collaborations [47−53] are involved in searching for heavy gauge bosons (such as
$ W{'} $ boson,$ Z{'} $ boson, and leptoquarks) from the proton-proton collisions at$ \sqrt{s}=13 $ TeV at LHC. By integrating theoretical principles with experimental findings, a range of phenomenological models for new physics (NP) have been constructed. These include$ {W}' $ model, non-universal$ {Z}' $ model, Higgs-doublet model, leptoquark model, and supersymmetry model. To explore the effects of NP through the consideration of$ W{'} $ boson and leptoquarks as intermediate particles, we examined$ c\to (s,d)\overline{l}{\nu }_{l} $ transitions in the framework of$ W{'} $ model and scalar leptoquark model. -
One of the beyond SM particle candidates is
$ {W}' $ boson which arises from the simplest extension of the electroweak gauge group$S U{\left(2\right)}_{1}\times S U{\left(2\right)}_{2}\times U\left(1\right)$ . In this model, we assumed that the exchanging of$ {W}' $ boson with the SM$ W $ boson causes the charged current transitions. Now the most general Lorentz invariant Lagrangian to describe the couplings of$ {W}'$ boson to quarks and leptons can be expressed as [14, 26, 27, 54, 55]:$ {\mathcal{L}}_{eff}^{{W}'}=\frac{{W}_{\mu }'}{\sqrt{2}}\left[{\overline{u}}_{i}\left({\epsilon}_{{u}_{i}{d}_{j}}^{L}{P}_{L}+{\epsilon}_{{u}_{i}{d}_{j}}^{R}{P}_{R}\right){\gamma }^{\mu }{d}_{j}+{\overline{l}}_{i}{\epsilon}_{{l}_{i}{v}_{j}}^{L}{\gamma }^{\mu }{P}_{L}{v}_{j}\right]+ {\rm h.c.} , $

(24) where
$ {P}_{L\left(R\right)}=(1\mp {\gamma }_{5})/2 $ denotes the left (right)-handed chirality projectors, and coefficients$ {\epsilon}_{{u}_{i}{d}_{j}}^{L} $ ,$ {\epsilon}_{{u}_{i}{d}_{j}}^{R} $ , and$ {\epsilon}_{{l}_{i}{v}_{j}}^{L} $ denote the dimensionless flavour-dependent coupling parameters with$ {u}_{i}\in \left(u,c,t\right),{d}_{j}\in (d,s,b) $ , and${l}_{i},{l}_{j}\in \left(e,\mu ,\tau \right).$ To simplify, the leptonic flavour dependent interactions ($ i=j $ ) have been considered in this study. In the SM,$ {\epsilon}_{{u}_{i}{d}_{j}}^{L}={g}_{L}{V}_{{u}_{i}{d}_{j}} $ and$ {\epsilon}_{{l}_{i}{v}_{i}}^{L}={g}_{L} $ where$ {g}_{L} $ denotes$S U{\left(2\right)}_{L}$ gauge coupling constant and$ {V}_{{u}_{i}{d}_{j}} $ denotes the corresponding CKM matrix element. In our model, the contribution of right-handed neutrino is ignored.For
$ c\to q\overline{l}{\nu }_{l} $ transition, the SM effective Lagrangian can be defined as:$ -{\mathcal{L}}_{\rm eff}{\left(c\to q\overline{l}{\nu }_{l}\right)}_{\rm SM}=\frac{4{G}_{F}{V}_{cq}}{\sqrt{2}}\left(\overline{q}{\gamma }^{\mu }{P}_{L}c\right)\left({\overline{\nu }}_{l}{\gamma }_{\mu }{P}_{L}l\right) . $

(25) From Eqs. (24) and (25), the total effective Lagrangian in
$ {W}' $ model can be expressed as:$ \begin{aligned}[b]&-{\mathcal{L}}_{\rm eff}{\left(c\to q\overline{l}{\nu }_{l}\right)}_{SM+{W}'}\\&=\;\frac{4{G}_{F}{V}_{cq}}{\sqrt{2}}\Bigg[\left(1+{{C}_{V}}_{L}\right)\left(\overline{q}{\gamma }^{\mu }{P}_{L}c\right)\left({\overline{\nu }}_{l}{\gamma }_{\mu }{P}_{L}l\right)\\&+{{C}_{V}}_{R}\left(\overline{q}{\gamma }^{\mu }{P}_{R}c\right)\left({\overline{\nu }}_{l}{\gamma }_{\mu }{P}_{L}l\right)\Bigg] , \end{aligned}$

(26) where
$ {{C}_{V}}_{L} $ and$ {{C}_{V}}_{R} $ denote the new Wilson coefficients corresponding to the left-handed and right-handed vector operator, respectively. Comparing Eq. (26) with Eq. (1), these Wilson coefficients can be expressed as [14, 26]:$ {C}_{{V}_{L}}\equiv \frac{\sqrt{2}}{4{G}_{F}{V}_{cq}}\frac{{\epsilon}_{qc}^{L}{\epsilon}_{{\nu }_{l}l}^{L}}{{M}_{{W}'}^{2}} $

$ {C}_{{V}_{R}}\equiv \frac{\sqrt{2}}{4{G}_{F}{V}_{cq}}\frac{{\epsilon}_{qc}^{R}{\epsilon}_{{\nu }_{l}l}^{L}}{{M}_{{W}'}^{2}} , $

(27) where
$ {M}_{{W}'} $ denotes the mass of$ {W}' $ boson,$ {\epsilon}_{qc}^{L,R} $ and$ {\epsilon}_{{\nu }_{l}l}^{L} $ denote the effective flavour dependent$ {W}' $ coupling parameters with quark and lepton, respectively.To examine production cross section of the hypothetical particle at 95% confidence level (CL), for a wide range of masses and couplings, experimental searches employed different models as benchmark scenarios. The coupling ranges have been set by several experimental studies to search for
$ {W}^{{'}} $ boson by considering the couplings of$ {W}' $ boson to be the same as those of SM$ W $ boson. This particular model is known as the sequential SM (SSM). The$ {W}' $ boson coupling strength$ {g}_{W'} $ is expressed in terms of the SM weak coupling strength${g}_{W}= {e}/{{\mathrm{sin}}^{2}{\theta }_{W}}=0.65.$ Hence, their coupling ratio is$ \dfrac{{g}_{{W}'}}{{g}_{W}}=1 $ in the SSM. The ATLAS and CMS collaboration have conducted search for this heavy boson in leptonic, semileptonic, and hadronic final states. They searched$ {W}' $ boson in$ e\nu $ and$ \mu \nu $ channels. ATLAS collaboration has set the lower limits of the$ {W}' $ boson mass at$ 6.0 $ TeV and$ 5.1 $ TeV in the electron and muon channels in the SSM scenario [48]. Using ATLAS [56] and CMS [57] data, Greljo et al. [58] fixed$ W{'} $ coupling parameter for the quark transition$ b\to c\tau {\overline{\nu }}_{\tau } $ with a$ {W}' $ mass range of 0.5−3.5 TeV. Gomez et al. [26] predicted the best-fitted value for this transition considering$ {M}_{{W}'}=1 $ TeV. Here, we considered the mass of$ {W}' $ boson$ \sim1 $ TeV and gauge coupling of$ {W}'$ boson identical to that of SM$ W $ boson. This is similar to SSM for experimental studies. Additionally, the couplings of this heavy boson to quark and lepton sectors are considered as having a non-universal nature in our proposed$ {W}' $ model.In this study, we adopted this model to study
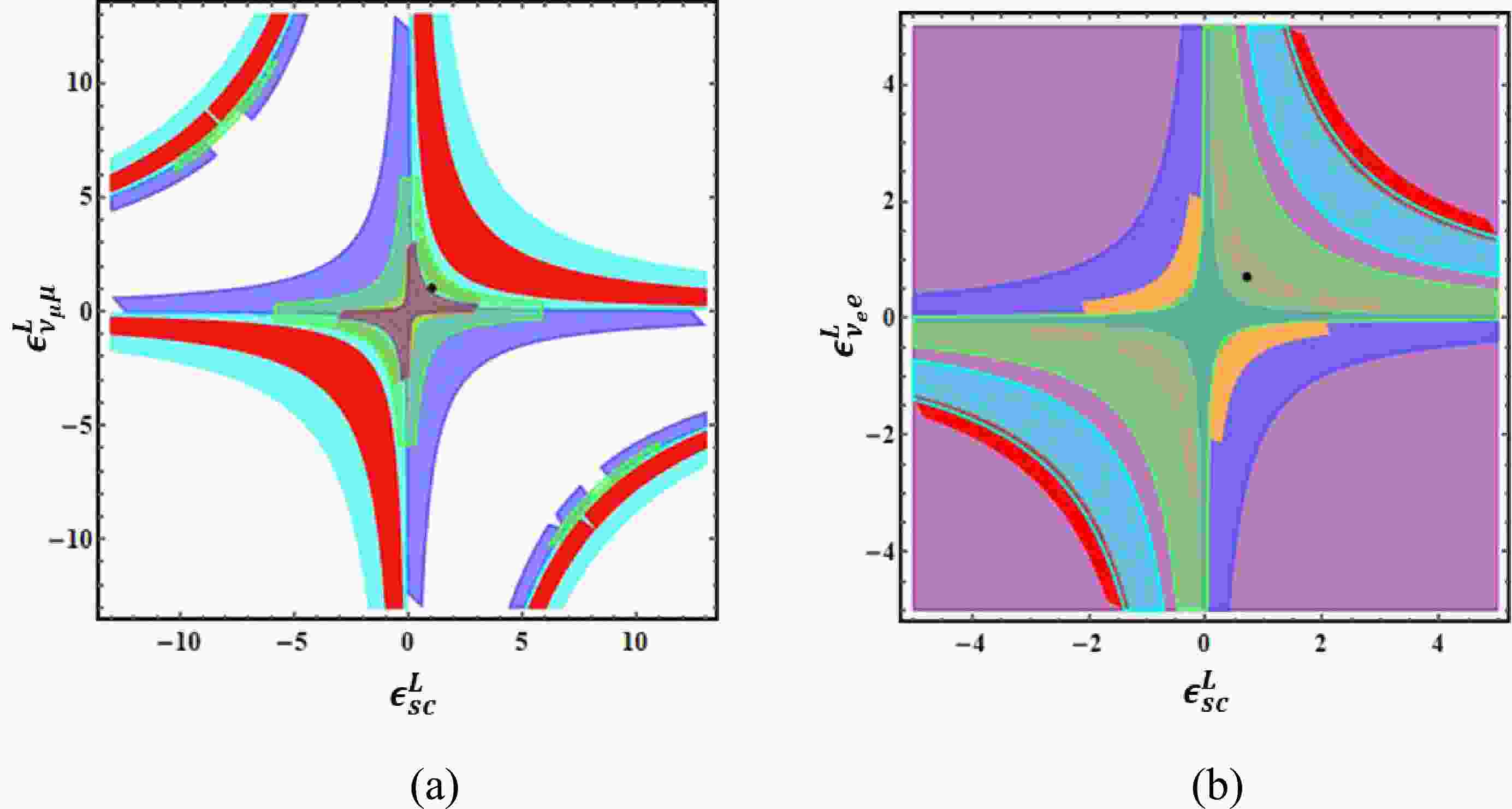
$ c\to (s,d)\overline{l}{\nu }_{l} $ transitions for the first time. Only the effect of left-handed$ {W}' $ boson has been considered in our model i.e.,$ {C}_{{V}_{R}}=0 $ , right-handed vector operator$ \left(\overline{q}{\gamma }^{\mu }{P}_{R}c\right) \times \left({\overline{\nu }}_{l}{\gamma }_{\mu }{P}_{L}l\right) $ does not contribute to the LFU violation at leading order [59]. New coupling parameters,$ {\epsilon}_{qc}^{L} $ and$ {\epsilon}_{{\nu }_{l}l}^{L} $ , have been fixed from the region plots (Figs. 1 and 2) using recent experimental results of branching fractions associated with$ c\to (s,d)\overline{l}{\nu }_{l} $ transitions, which are summarized in Table 3.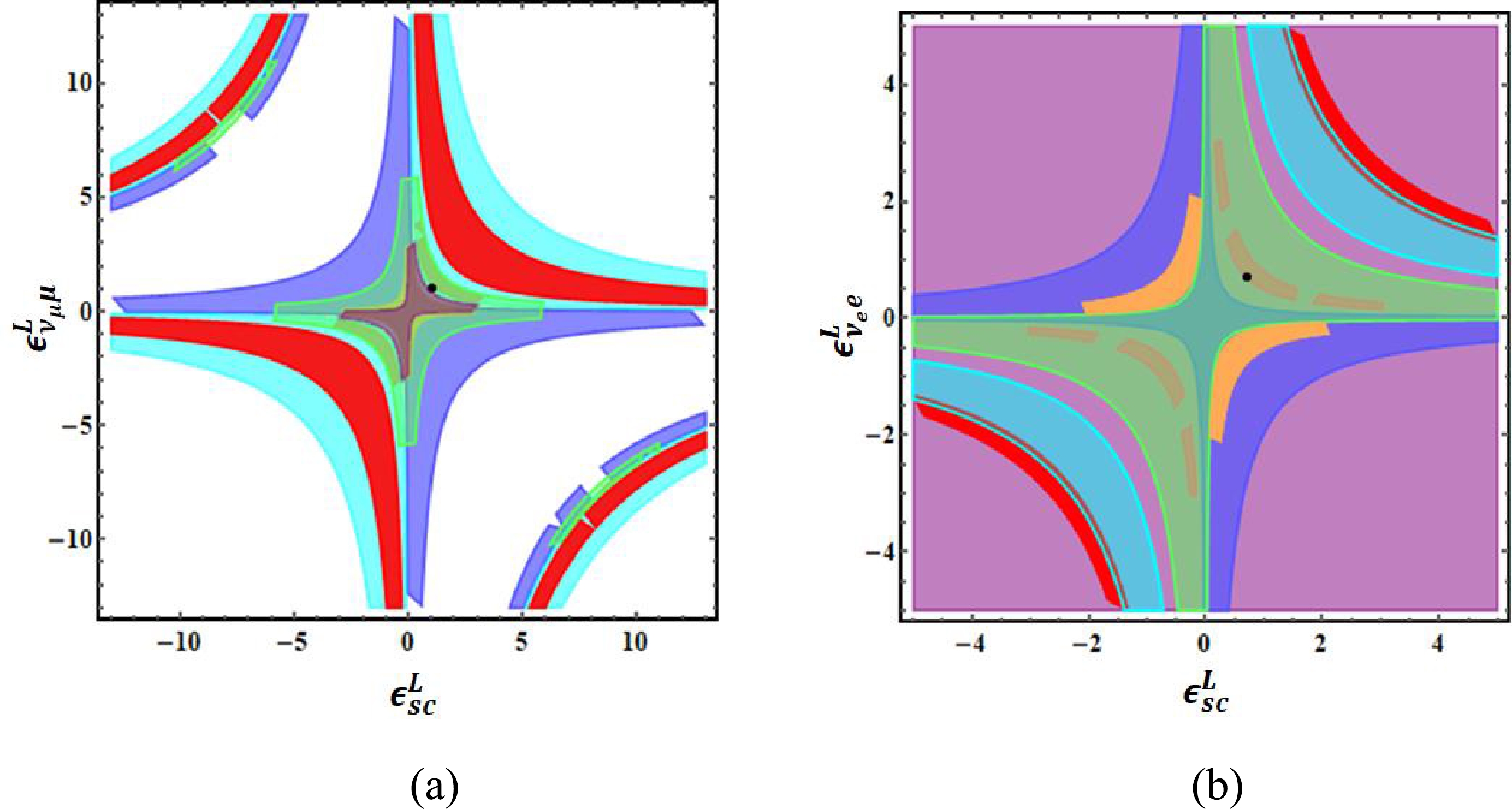
Figure 1. (color online) Allowed parameter space in (
$ {\epsilon}_{sc}^{L} $ ,$ {\epsilon}_{{\nu }_{l}l}^{L} $ ) plane for (a)$ c\to s{\mu }^{+}{\nu }_{\mu } $ and (b)$ c\to s{e}^{+}{\nu }_{e} $ transitions. Here, colour notations are: Red$ ({D}_{s}^{+}\to \eta $ ), Cyan ($ {D}_{s}^{+}\to {\eta }{{'}} $ ), Brown ($ {D}^{+}\to {\overline{K}}^{*0} $ ), Blue ($ {D}_{s}^{+}\to \varphi $ ), Green ($ {D}^{0}\to {K}^{*-} $ ), Orange ($ {D}^{+}\to {\overline{K}}^{0} $ and$ {D}^{0}\to {K}^{-} $ ), and Purple ($ {D}_{s}^{+}\to {l}^{+}{\nu }_{l} $ ). Black dot represents the fitted point of coupling parameters.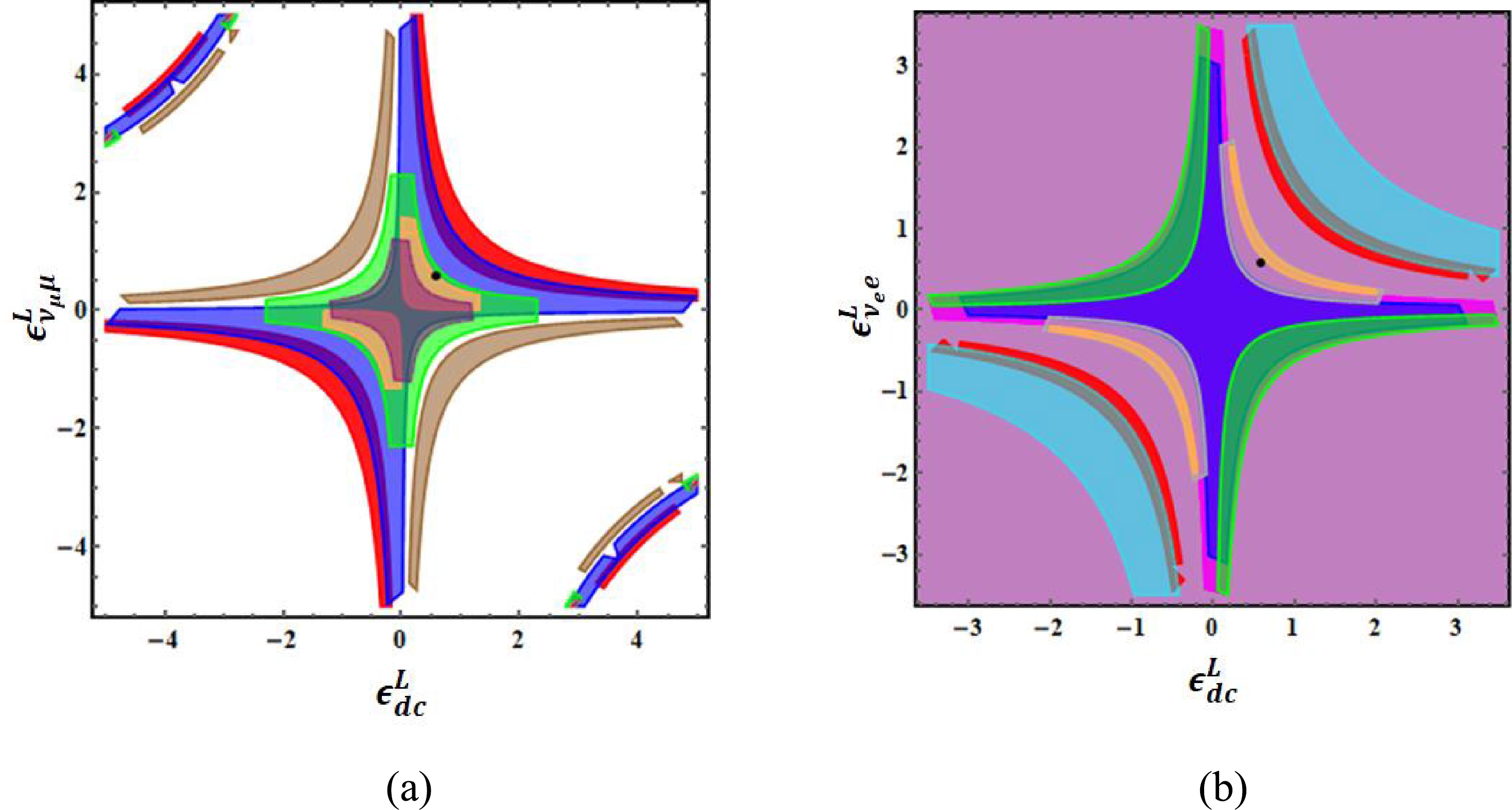
Figure 2. (color online) Allowed parameter space in (
$ {\epsilon}_{dc}^{L} $ ,$ {\epsilon}_{{\nu }_{l}l}^{L} $ ) plane for (a)$ c\to d{\mu }^{+}{\nu }_{\mu } $ and (b)$ c\to d{e}^{+}{\nu }_{e} $ transitions. Here, colour notations are: Red$ ({D}^{+}\to \eta $ ), Cyan ($ {D}^{+}\to {\eta }{{'}} $ ), Blue ($ {D}^{+}\to {\rho }^{0} $ ), Green ($ {D}^{+}\to {\omega }^{0} $ ), Brown ($ {D}^{0}\to {\rho }^{-} $ ), Magenta ($ {D}_{s}^{+}\to {K}^{*0} $ ), Gray ($ {D}^{+}\to {\pi }^{0} $ ), Orange ($ {D}^{0}\to {\pi }^{-} $ ), and Purple ($ {D}^{+}\to {l}^{+}{\nu }_{l} $ ). Black dot represents the fitted point of coupling parameters.Current Coupling Parameter Mid value $ c\to s{\mu }^{+}{\nu }_{\mu } $ 

$ {\epsilon}_{sc}^{L}{\epsilon}_{{\nu }_{\mu }\mu }^{L} $ 

$ 1.01 $ 

$ c\to s{e}^{+}{\nu }_{e} $ 

$ {\epsilon}_{sc}^{L}{\epsilon}_{{\nu }_{e}e}^{L} $ 

$ 0.49 $ 

$ c\to d{\mu }^{+}{\nu }_{\mu } $ 

$ {\epsilon}_{dc}^{L}{\epsilon}_{{\nu }_{\mu }\mu }^{L} $ 

$ 0.35 $ 

$ c\to d{e}^{+}{\nu }_{e} $ 

$ {\epsilon}_{dc}^{L}{\epsilon}_{{\nu }_{e}e}^{L} $ 

$ 0.34 $ 

Table 3. Values of the coupling parameters in
$ W{'} $ model. -
Beyond SM, quarks can transform into leptons and vice versa through the intermediate hypothetical particles termed as leptoquarks (LQ). This is possible through grand unification theories (GUT) in which matter and forces are unified. In these theories, the transformation between quarks and leptons has a higher-order symmetry multiplet. Recently, the search for LQ in both theory and experiments has become more feasible for extending the SM. [60–63]. Leptoquarks exhibit both spin zero (
$ s=0 $ ) – scalar leptoquarks and one ($ s=1 $ ) – vector leptoquarks, they also carry colour and fractional electric charge. Experimental searches for LQs at colliders have been conduted by considering the same couplings to all SM fermions or enhanced couplings to second- or third-generation fermions. Based on the$ pp\to \tau \nu $ process, which is mediated by exchange of LQ, lower limits on the masses of LQ are set at$205/515/5900\;\mathrm{G}\mathrm{e}\mathrm{V}$ for the best fit LH / best-fit LH+RH / democratic scenarios by CMS collaboration [50]. From the significant part of the LQ parameter space, CMS have probed for LQ with masses up to$10\;\mathrm{T}\mathrm{e}\mathrm{V}$ and LQ couplings of up to 8. The LQ mass has been set in the range of$ 0.98-1.73 $ TeV, depending on the LQ spin and its coupling to a lepton and quark [51]. We considered an LQ mass of$ ~1 $ TeV in our scalar LQ model, and the coupling to the quarks and leptons have been considered as non-universal. Considering mass${M}_{\varphi }\approx 1$ TeV and$ O\left(1\right) $ coupling, a NP model is developed in the Ref. [63] which can explain$ {R}_{{D}^{*}} $ anomalies. To describe the transformation between quark and lepton through LQ as mediator, the Lagrangian can be defined as [14, 64]:$ {\mathcal{L}}_{int}^{\phi }\supset {\overline{Q}}_{L}^{c}{\lambda }^{L}i{\tau }_{2}L{\phi }^{*}+{\overline{u}}_{R}^{c}{\lambda }^{R}{l}_{R}{\varphi }^{*}+ {\rm h.c.}, $

(28) where
$ {Q}_{L} $ and$ L $ denote the left-handed quark and lepton doublet, respectively, and$ {u}_{R} $ and$ {l}_{R} $ denote the right-handed up-type quark and lepton singlet, respectively. The Yukawa coupling matrices in flavour space are denoted by$ {\lambda }^{L,R} $ . The basic key feature of the model is that the$ c\to q\overline{l}{\nu }_{l} $ transition occurs through vector ($ {O}_{{V}_{L}} $ ), scalar ($ {O}_{{S}_{L}} $ ), and tensor ($ {O}_{T} $ ) currents by intermediate scalar LQ$ \varphi $ , and their corresponding Wilson coefficients are defined as [14]:$\begin{aligned}[b]& {C}_{{V}_{L}}\left({M}_{\varphi }\right)=\frac{{\lambda }_{c{\nu }_{l}}^{L}{\lambda }_{ql}^{L*}}{4\sqrt{2}{G}_{F}{V}_{cq}{M}_{\varphi }^{2}} , \\&{C}_{{S}_{L}}\left({M}_{\varphi }\right)=-\frac{{\lambda }_{c{\nu }_{l}}^{L}{\lambda }_{ql}^{R*}}{4\sqrt{2}{G}_{F}{V}_{cq}{M}_{\varphi }^{2}} ,\\& {C}_{T}\left({M}_{\varphi }\right)=-\frac{1}{4}{S}_{L}\left({M}_{\varphi }\right) .\end{aligned} $

(29) Using recent experimental results of branching fractions provided in Tables 4 and 5 and setting
$ {M}_{\varphi }=1 $ TeV, the new Yukawa couplings are predicted from the region plots shown in Figs. 3 and 4. The results are summarized in Table 6. We considered 10% uncertainty of the coupling parameters for our study.Decay SM result [18] Exp. result [24] $ {D}^{+}\to {e}^{+}{\nu }_{e} $ 

$ (9.17\pm 0.22)\times {10}^{-9} $ 

$ < 8.8\times {10}^{-6} $ 

$ {D}^{+}\to {\mu }^{+}{\nu }_{\mu } $ 

$ (3.89\pm 0.09)\times {10}^{-4} $ 

$ (3.74\pm 0.17)\times {10}^{-4} $ 

$ {D}_{s}^{+}\to {e}^{+}{\nu }_{e} $ 

$ (1.24\pm 0.02)\times {10}^{-7} $ 

$ < 8.3\times {10}^{-5} $ 

$ {D}_{s}^{+}\to {\mu }^{+}{\nu }_{\mu } $ 

$ (5.28\pm 0.08)\times {10}^{-3} $ 

$ (5.43\pm 0.15)\times {10}^{-3} $ 

Table 4. SM prediction of branching fractions for leptonic
$ D\left({D}_{s}\right) $ decay and corresponding experimental results.$ c\to s{\mu }^{+}{\nu }_{\mu } $ 

Decay SM Result [18] Exp. Result [24] $ {D}^{0}\to {K}^{-}{\mu }^{+}{\nu }_{\mu } $ 

$ (3.40\pm 0.22)\times {10}^{-2} $ 

$ (3.41\pm 0.04)\times {10}^{-2} $ 

$ {D}^{+}\to {\stackrel{-}{K}}^{0}{\mu }^{+}{\nu }_{\mu } $ 

$ (8.69\pm 0.57)\times {10}^{-2} $ 

$ (8.76\pm 0.19)\times {10}^{-2} $ 

$ {D}^{0}\to {K}^{*-}{\mu }^{+}{\nu }_{\mu } $ 

$ (1.81\pm 0.16)\times {10}^{-2} $ 

$ (1.89\pm 0.24)\times {10}^{-2} $ 

$ {D}^{+}\to {\stackrel{-}{K}}^{*0}{\mu }^{+}{\nu }_{\mu } $ 

$ (4.71\pm 0.42)\times {10}^{-2} $ 

$ (5.27\pm 0.15)\times {10}^{-2} $ 

$ {D}_{s}^{+}\to \varphi {\mu }^{+}{\nu }_{\mu } $ 

$ (2.33\pm 0.40)\times {10}^{-2} $ 

$ (1.90\pm 0.50)\times {10}^{-2} $ 

$ {D}_{s}^{+}\to \eta {\mu }^{+}{\nu }_{\mu } $ 

$ (1.52\pm 0.31)\times {10}^{-2} $ 

$ (2.4\pm 0.5)\times {10}^{-2} $ 

$ {D}_{s}^{+}\to {\eta }^{{'}}{\mu }^{+}{\nu }_{\mu } $ 

$ (5.64\pm 1.10)\times {10}^{-3} $ 

$ (11.0\pm 5.0)\times {10}^{-3} $ 

$ c\to s{e}^{+}{\nu }_{e} $ 

Decay SM Result [18] Exp. Result [24] $ {D}^{0}\to {K}^{-}{e}^{+}{\nu }_{e} $ 

$ (3.49\pm 0.23)\times {10}^{-2} $ 

$ (3.542\pm 0.0035)\times {10}^{-2} $ 

$ {D}^{+}\to {\stackrel{-}{K}}^{0}{e}^{+}{\nu }_{e} $ 

$ (8.92\pm 0.59)\times {10}^{-2} $ 

$ (8.73\pm 0.10)\times {10}^{-2} $ 

$ {D}^{0}\to {K}^{*-}{e}^{+}{\nu }_{e} $ 

$ (1.92\pm 0.17)\times {10}^{-2} $ 

$ (2.15\pm 0.16)\times {10}^{-2} $ 

$ {D}^{+}\to {\stackrel{-}{K}}^{*0}{e}^{+}{\nu }_{e} $ 

$ (4.98\pm 0.45)\times {10}^{-2} $ 

$ (5.40\pm 0.10)\times {10}^{-2} $ 

$ {D}_{s}^{+}\to \varphi {e}^{+}{\nu }_{e} $ 

$ (2.46\pm 0.42)\times {10}^{-2} $ 

$ (2.39\pm 0.16)\times {10}^{-2} $ 

$ {D}_{s}^{+}\to \eta {e}^{+}{\nu }_{e} $ 

$ (1.55\pm 0.33)\times {10}^{-2} $ 

$ (2.29\pm 0.19)\times {10}^{-2} $ 

$ {D}_{s}^{+}\to {\eta }^{{'}}{e}^{+}{\nu }_{e} $ 

$ (5.91\pm 1.26)\times {10}^{-3} $ 

$ (7.4\pm 1.4)\times {10}^{-3} $ 

$ c\to d{\mu }^{+}{\nu }_{\mu } $ 

Decay SM Result [18] Exp. Result [24] $ {D}^{0}\to {\pi }^{-}{\mu }^{+}{\nu }_{\mu } $ 

$ (2.60\pm 0.31)\times {10}^{-3} $ 

$ (2.67\pm 0.12)\times {10}^{-3} $ 

$ {D}^{+}\to {\pi }^{0}{\mu }^{+}{\nu }_{\mu } $ 

$ (3.37\pm 0.40)\times {10}^{-3} $ 

$ (3.50\pm 0.15)\times {10}^{-3} $ 

$ {D}^{0}\to {\rho }^{-}{\mu }^{+}{\nu }_{\mu } $ 

$ (1.65\pm 0.23)\times {10}^{-3} $ 

$ (1.35\pm 0.13)\times {10}^{-3} $ 

$ {D}^{+}\to {\rho }^{0}{\mu }^{+}{\nu }_{\mu } $ 

$ (2.14\pm 0.30)\times {10}^{-3} $ 

$ (2.4\pm 0.4)\times {10}^{-3} $ 

$ {D}^{+}\to {\omega }^{0}{\mu }^{+}{\nu }_{\mu } $ 

$ (1.82\pm 0.26)\times {10}^{-3} $ 

$ (1.77\pm 0.21)\times {10}^{-3} $ 

$ {D}^{+}\to \eta {\mu }^{+}{\nu }_{\mu } $ 

$ (0.75\pm 0.15)\times {10}^{-3} $ 

$ (1.04\pm 0.11)\times {10}^{-3} $ 

$ c\to d{e}^{+}{\nu }_{e} $ 

Decay SM Result [18] Exp. Result [24] $ {D}^{0}\to {\pi }^{-}{e}^{+}{\nu }_{e} $ 

$ (2.63\pm 0.32)\times {10}^{-3} $ 

$ (2.91\pm 0.04)\times {10}^{-3} $ 

$ {D}^{+}\to {\pi }^{0}{e}^{+}{\nu }_{e} $ 

$ (3.41\pm 0.41)\times {10}^{-3} $ 

$ (3.72\pm 0.17)\times {10}^{-3} $ 

$ {D}_{s}^{+}\to {K}^{*0}{e}^{+}{\nu }_{e} $ 

$ (2.33\pm 0.34)\times {10}^{-3} $ 

$ (2.15\pm 0.28)\times {10}^{-3} $ 

$ {D}^{0}\to {\rho }^{-}{e}^{+}{\nu }_{e} $ 

$ (1.74\pm 0.25)\times {10}^{-3} $ 

$ (1.50\pm 0.12)\times {10}^{-3} $ 

$ {D}^{+}\to {\rho }^{0}{e}^{+}{\nu }_{e} $ 

$ (2.25\pm 0.32)\times {10}^{-3} $ 

$ \left({2.18}_{-0.25}^{+0.17}\right)\times {10}^{-3} $ 

$ {D}^{+}\to {\omega }^{0}{e}^{+}{\nu }_{e} $ 

$ (1.91\pm 0.27)\times {10}^{-3} $ 

$ (1.69\pm 0.11)\times {10}^{-3} $ 

$ {D}^{+}\to \eta {e}^{+}{\nu }_{e} $ 

$ (0.76\pm 0.16)\times {10}^{-3} $ 

$ (1.11\pm 0.07)\times {10}^{-3} $ 

$ {D}^{+}\to {\eta }^{{'}}{e}^{+}{\nu }_{e} $ 

$ (1.12\pm 0.24)\times {10}^{-4} $ 

$ (2.0\pm 0.4)\times {10}^{-4} $ 

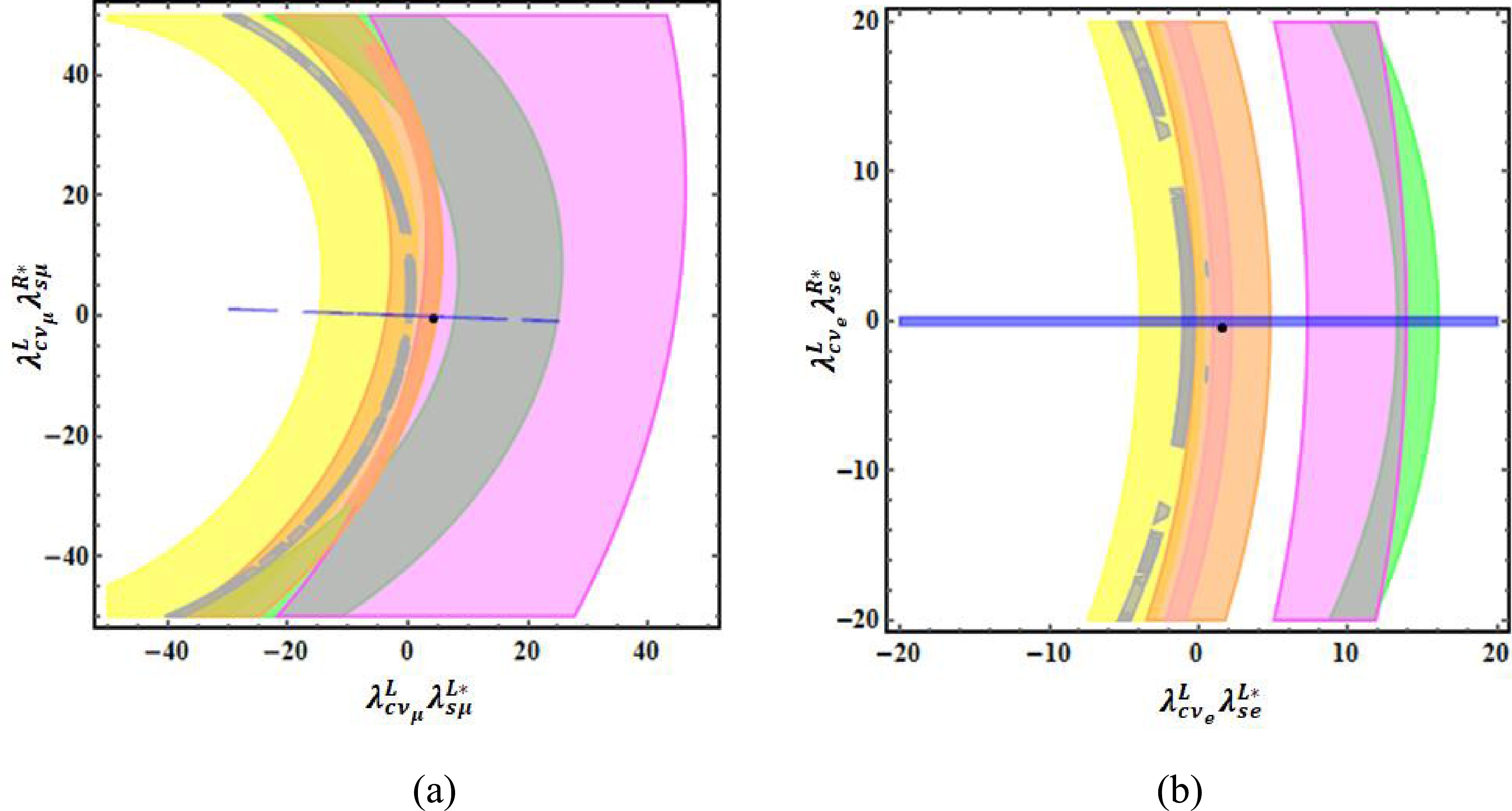
Figure 3. (color online) Allowed parameter space in (
$ {\lambda }_{c{\nu }_{l}}^{L}{\lambda }_{sl}^{{L}^{*}} $ ,$ {\lambda }_{c{\nu }_{l}}^{L}{\lambda }_{sl}^{{R}^{*}} $ ) plane for (a)$ c\to s{\mu }^{+}{\nu }_{\mu } $ and (b)$ c\to s{e}^{+}{\nu }_{e} $ transitions. Here, colour notations are: Green$ ({D}_{s}^{+}\to \eta $ ), Magenta ($ {D}_{s}^{+}\to {\eta'} $ ), Yellow ($ {D}_{s}^{+}\to \varphi $ ), Pink ($ {D}^{+}\to {\bar{K}}^{*0} $ ), Orange ($ {D}^{0}\to {K}^{*-} $ ), Grey ($ {D}^{0}\to {K}^{-} $ and$ {D}^{+}\to {\bar{K}}^{0} $ ), and Blue ($ {D}_{s}^{+}\to {l}^{+}{\nu }_{l} $ ). Black dot represents the fitted point of coupling parameters.
Figure 4. (color online) Allowed parameter space in (
$ {\lambda }_{c{\nu }_{l}}^{L}{\lambda }_{dl}^{{L}^{*}} $ ,$ {\lambda }_{c{\nu }_{l}}^{L}{\lambda }_{dl}^{{R}^{*}} $ ) plane for (a)$ c\to d{\mu }^{+}{\nu }_{\mu } $ and (b)$ c\to d{e}^{+}{\nu }_{e} $ transitions. Here, colour notations are: Green$ ({D}^{+}\to \eta $ ), Magenta ($ {D}^{+}\to {\eta }{{'}} $ ), Blue ($ {D}^{+}\to \omega $ ), Cyan ($ {D}^{0}\to {\rho }^{-} $ ), Red ($ {D}^{+}\to {\rho }^{0} $ ), Yellow ($ {D}_{s}^{+}\to {K}^{0-} $ ), Brown ($ {D}^{0}\to {\pi }^{-} $ and$ {D}^{+}\to {\pi }^{0} $ ), and Purple ($ {D}^{+}\to {l}^{+}{\nu }_{l} $ ). Black dot represents the fitted point of coupling parameters.Current Coupling Parameter Mid value $ c\to s{\mu }^{+}{\nu }_{\mu } $ 

$ {\lambda }_{c{\nu }_{\mu }}^{L}{\lambda }_{s\mu }^{L*} $ 

$ 4.30 $ 

$ {\lambda }_{c{\nu }_{\mu }}^{L}{\lambda }_{s\mu }^{R*} $ 

$ -0.47 $ 

$ c\to s{e}^{+}{\nu }_{e} $ 

$ {\lambda }_{c{\nu }_{e}}^{L}{\lambda }_{se}^{L*} $ 

$ 1.63 $ 

$ {\lambda }_{c{\nu }_{e}}^{L}{\lambda }_{se}^{R*} $ 

$ -0.44 $ 

$ c\to d{\mu }^{+}{\nu }_{\mu } $ 

$ {\lambda }_{c{\nu }_{\mu }}^{L}{\lambda }_{d\mu }^{L*} $ 

$ 0.78 $ 

$ {\lambda }_{c{\nu }_{\mu }}^{L}{\lambda }_{d\mu }^{R*} $ 

$ -0.05 $ 

$ c\to d{e}^{+}{\nu }_{e} $ 

$ {\lambda }_{c{\nu }_{e}}^{L}{\lambda }_{de}^{L*} $ 

$ 1.0 $ 

$ {\lambda }_{c{\nu }_{e}}^{L}{\lambda }_{de}^{R*} $ 

$ -0.18 $ 

Table 6. Values of the scalar LQ coupling parameters.
-
In this study, we examine
$ {D}_{\left(s\right)}^{+}\to {\eta }^{\left({'}\right)}\overline{l}{\nu }_{l} $ decays involving$ c\to (s,d)\overline{l}{\nu }_{l} $ transitions in the aforementioned two NP models. First, we structured these two models. Then, all the necessary coupling parameters are predicted from region plots using recent experimental results of branching fractions summarized in Tables 4 and 5. Predicted coupling parameters are listed in Tables 3 and 6. The hadronic form factors are one of the key input parameters for this theoretical study. To date, LQCD results are unavailable. Hence, we used the results calculated by LCSR in our calculation which are presented in Table 2. The values of other input parameters are also summarized in the appendix.Decay $ D\to {\eta }_{q} $ 

$ {D}_{s}\to {\eta }_{s} $ 

Form factors $ {f}_{+} $ 

$ {f}_{0} $ 

$ {f}_{+} $ 

$ {f}_{0} $ 

$ F\left(0\right) $ 

$ {0.56}_{-0.05}^{+0.06} $ 

$ {0.56}_{-0.05}^{+0.06} $ 

$ {0.61}_{-0.05}^{+0.06} $ 

$ {0.61}_{-0.05}^{+0.06} $ 

$ a $ 

$ {1.25}_{+0.05}^{-0.04} $ 

$ {0.65}_{+0.02}^{-0.01} $ 

$ {1.20}_{+0.03}^{-0.02} $ 

$ {0.64}_{+0.02}^{-0.01} $ 

$ b $ 

$ {0.42}_{+0.05}^{-0.06} $ 

$ {-0.22}_{+0.02}^{-0.03} $ 

$ {0.38}_{+0.01}^{-0.01} $ 

$ {-0.18}_{-0.03}^{+0.04} $ 

Using
$ {W}' $ model, we mainly investigated differential branching fraction for the aforementioned decays. The$ {q}^{2} $ dependence of differential branching fractions have been depicted in Figs. 5 and 6. The significant contributions of$ {W}' $ boson has been observed in all decays except$ {D}_{s}^{+}\to {\eta }^{\left({'}\right)}{e}^{+}{\nu }_{e} $ decays. All the results of branching fractions are provided in Table 7, and results using this model determined approximately$ 1.5\sigma $ range of the experimental results. Basically$ {W}' $ model has NP contribution through the left-handed vector Willson coefficient ($ {C}_{{V}_{L}} $ ) corresponding to the vector operator ($ {O}_{{V}_{L}} $ ). The$ {C}_{{V}_{L}} $ neutralizes the other two observables forward-backward asymmetry$ {A}_{FB}\left({q}^{2}\right) $ and lepton polarization asymmetry$ {P}_{F}^{l}\left({q}^{2}\right) $ . Therefore, this model is unable to provide NP in these decays and it is only suitable to study branching fraction.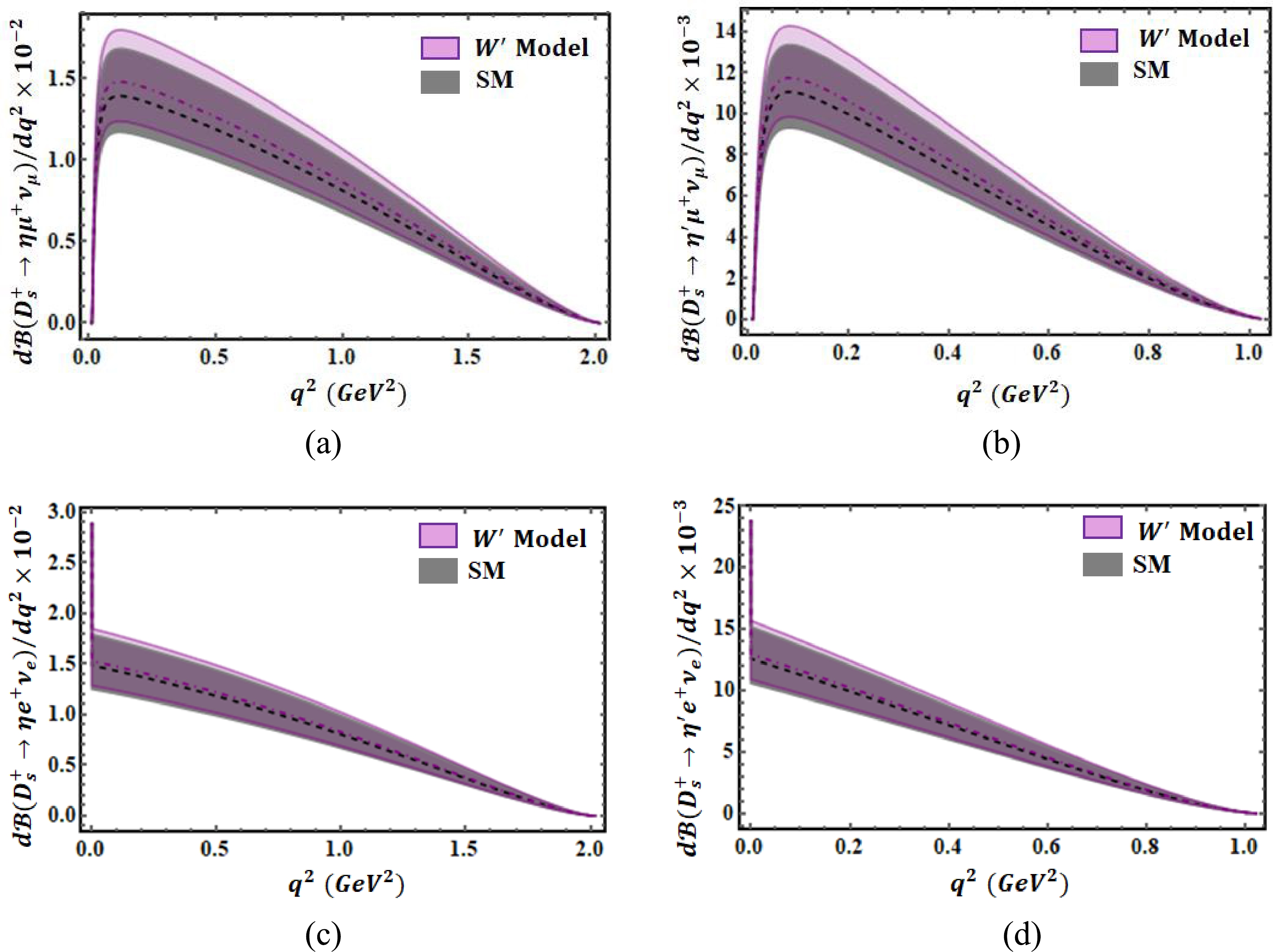
Figure 5. (color online)
$ {q}^{2} $ dependence of branching fractions for$ {D}_{s}^{+}\to {\eta }^{\left({'}\right)}\bar{l}{\nu }_{l} $ in SM and$ {W}' $ model. Specifically, black dashed and purple dash-doted lines present the central variation in SM and$ W{'} $ model, respectively.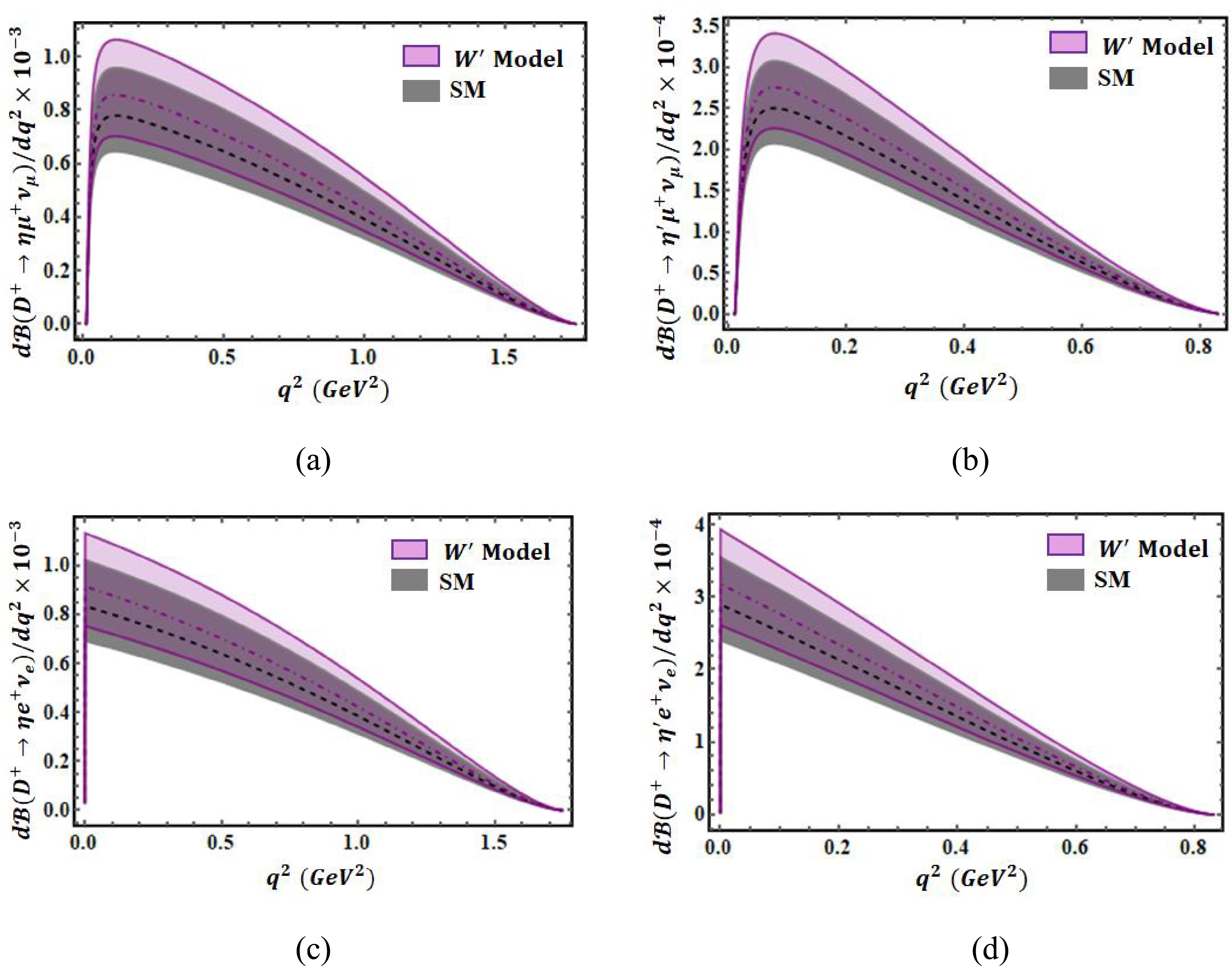
Figure 6. (color online)
$ {q}^{2} $ dependence of branching fractions for${D}^{+}\to {\eta }^{\left({'}\right)}\bar{l}{\nu }_{l}$ in SM and$ {W}' $ model. Specifically, black dashed and purple dash-doted lines present the central variation in SM and$ W{'} $ model, respectively.Decay $ {W}' $ model

Scalar LQ model Experiment [24] $ Pul{l}_{SM} $ 

$ Pul{l}_{{W}'} $ 

$ Pul{l}_{LQ} $ 

$ \mathcal{B}({D}_{s}^{+}\to \eta {\mu }^{+}{\nu }_{\mu })\times {10}^{-2} $ 

$ 1.61\pm 0.37 $ 

$ 1.73\pm 0.41 $ 

$ 2.4\pm 0.5 $ 

$ 1.5\sigma $ 

$ 1.3\sigma $ 

$ 1.0\sigma $ 

$ 2.215\pm 0.051\pm 0.052 $ [25]

$ 2.2\sigma $ 

$ 1.6\sigma $ 

$ 1.1\sigma $ 

$ {\mathcal{B}(D}_{s}^{+}\to {\eta }{{'}}{\mu }^{+}{\nu }_{\mu })\times {10}^{-3} $ 

$ 6.00\pm 1.31 $ 

$ 6.42\pm 1.47 $ 

$ 11.0\pm 5.0 $ 

$ 1.05\sigma $ 

$ 0.9\sigma $ 

$ 0.9\sigma $ 

$ 8.01\pm 0.55\pm 0.31 $ [25]

$ 1.9\sigma $ 

$ 1.3\sigma $ 

$ 0.8\sigma $ 

$ {\mathcal{B}(D}_{s}^{+}\to \eta {e}^{+}{\nu }_{e})\times {10}^{-2} $ 

$ 1.59\pm 0.36 $ 

$ 1.62\pm 0.38 $ 

$ 2.29\pm 0.19 $ 

$ 2\sigma $ 

$ 1.7\sigma $ 

$ 1.5\sigma $ 

$ {\mathcal{B}(D}_{s}^{+}\to {\eta }{{'}}{e}^{+}{\nu }_{e})\times {10}^{-3} $ 

$ 6.09\pm 1.32 $ 

$ 6.21\pm 1.36 $ 

$ 7.4\pm 1.4 $ 

$ 0.8\sigma $ 

$ 0.7\sigma $ 

$ 0.6\sigma $ 

$ {\mathcal{B}(D}^{+}\to \eta {\mu }^{+}{\nu }_{\mu })\times {10}^{-3} $ 

$ 0.82\pm 0.21 $ 

$ 0.83\pm 0.21 $ 

$ 1.04\pm 0.11 $ 

$ 1.6\sigma $ 

$ 0.9\sigma $ 

$ 0.9\sigma $ 

$ {\mathcal{B}(D}^{+}\to {\eta }{{'}}{\mu }^{+}{\nu }_{\mu })\times {10}^{-4} $ 

$ 1.15\pm 0.28 $ 

$ 1.16\pm 0.29 $ 

− − − − $ {\mathcal{B}(D}^{+}\to \eta {e}^{+}{\nu }_{e})\times {10}^{-3} $ 

$ 0.83\pm 0.22 $ 

$ 0.87\pm 0.23 $ 

$ 1.11\pm 0.07 $ 

$ 2\sigma $ 

$ 1.2\sigma $ 

$ 0.9\sigma $ 

$ {\mathcal{B}(D}^{+}\to {\eta }{{'}}{e}^{+}{\nu }_{e})\times {10}^{-4} $ 

$ 1.21\pm 0.30 $ 

$ 1.26\pm 0.31 $ 

$ 2.0\pm 0.4 $ 

$ 1.9\sigma $ 

$ 1.6\sigma $ 

$ 1.5\sigma $ 

Table 7. Values of the branching fractions in
$ W{'} $ model and scalar LQ model and their corresponding pull from the experimental results.Next, we have focused on scalar LQ model. It provides NP effect through the vector, scalar, and tensor operators. Therefore, it is capable of examining NP dependent decay observables for finding potential NP signal. In this study, differential branching fraction, forward-backward asymmetry, and lepton polarization asymmetry have been investigated in this model. In Figs. 7 and 8,
$ {q}^{2} $ dependence of branching fractions have been shown. The significant effects of scalar LQ model on diffentical branching fraction have been observed in all decays. Using this model, our predicted values of branching fractions and their corresponding pulls from the experimental results are provided in Table 7. All results are based around the$ 1\sigma $ range of the experimental results excludeing$ {D}_{s}^{+}\to \eta {e}^{+}{\nu }_{e} $ and$ {D}^{+}\to {\eta }{{'}}{e}^{+}{\nu }_{e} $ decays. Hence, this model successfully explains all of the experimental findings bettern than$ W{'} $ model. Any contribution of scalar LQ is not observed for the forward-backward asymmetry and lepton polarization asymmetry. In the NP framework, these two decay observables mainly depend on the NP Wilson coefficient$ {C}_{T} $ , and this equals to the one fourth of$ {C}_{{S}_{L}} $ . By putting all the values in Eq. (29), we obtain$ {C}_{{S}_{L}}=0.7\times {10}^{-2} $ and$ {C}_{T}=0.2\times {10}^{-2} $ for the$ c\to s{\mu }^{+}{\nu }_{\mu } $ transition. Given the very small value of$ {C}_{T} $ , a significant effect of scalar LQ on these obsevables has not been found in scalar LQ model.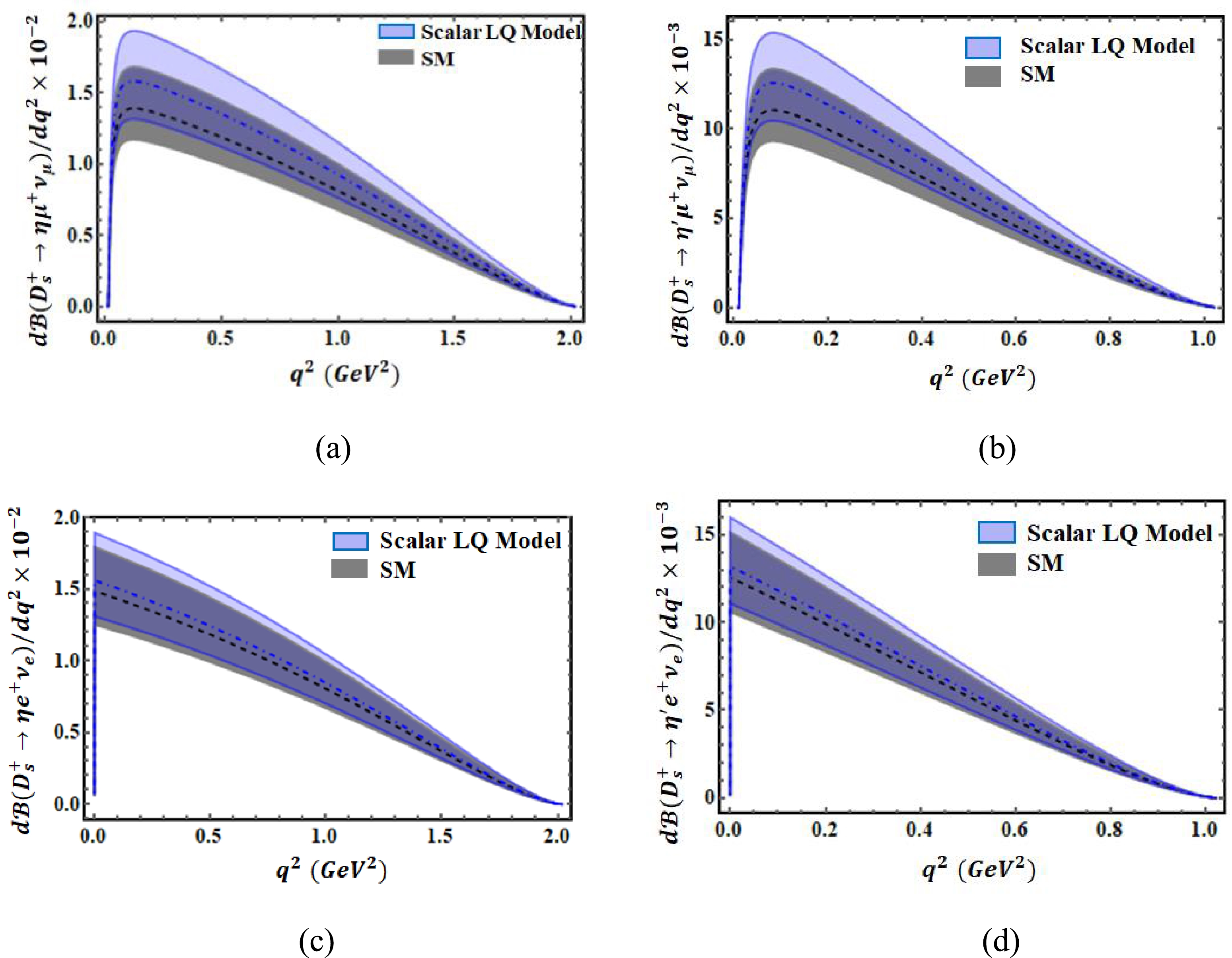
Figure 7. (color online)
$ {q}^{2} $ dependence of branching fractions for$ {D}_{s}^{+}\to {\eta }^{\left({'}\right)}\bar{l}{\nu }_{l} $ decays in SM and scalar LQ model. Specifically, black dashed and blue dash-doted lines denote the central variation in SM and scalar LQ model, respectively.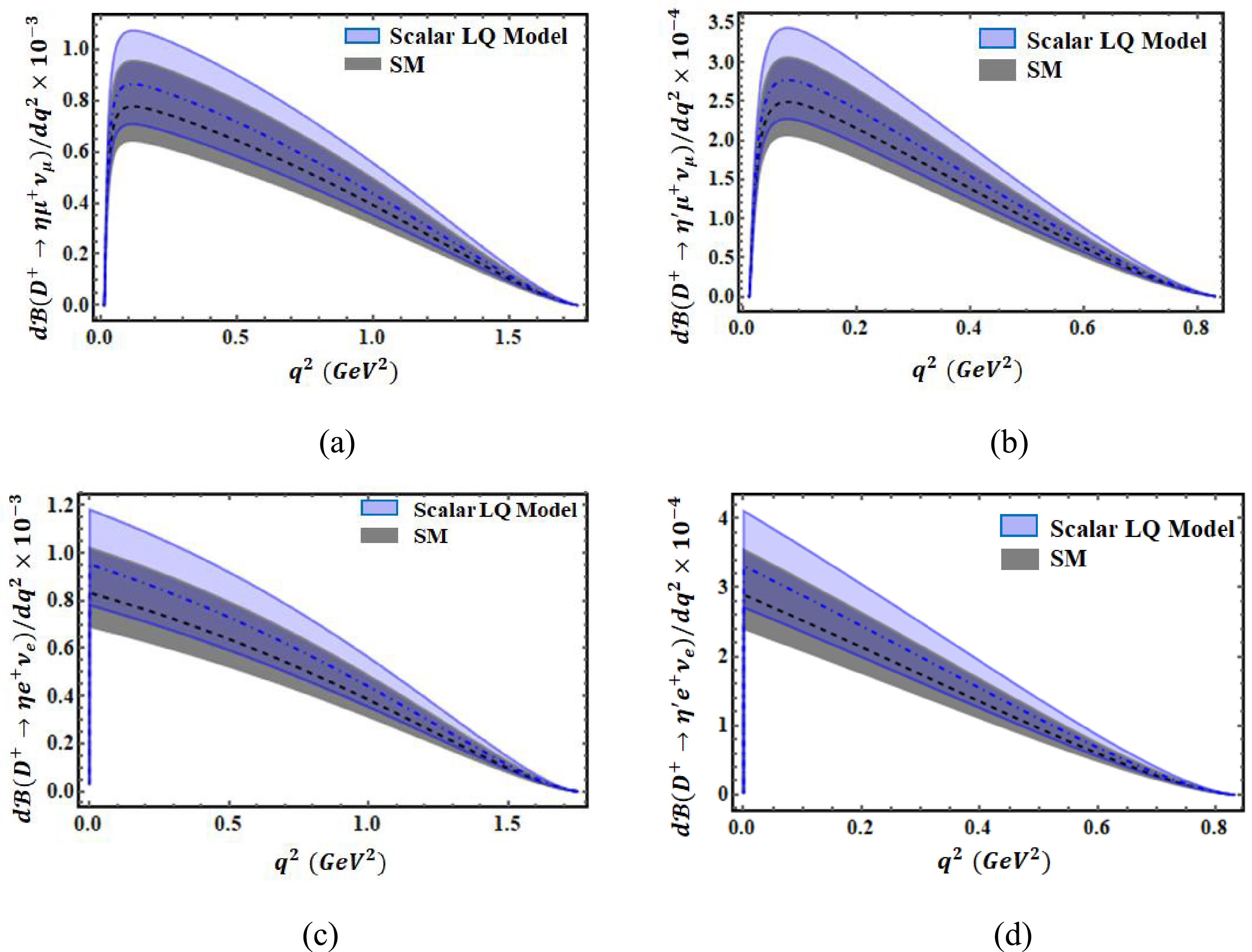
Figure 8. (color online)
$ {q}^{2} $ dependence of branching fractions for${D}^{+}\to {\eta }^{\left({'}\right)}\bar{l}{\nu }_{l}$ decays in SM and Scalar LQ model. Specifically, black dashed and blue dash-doted lines present the central variation in SM and scalar LQ model, respectively.Finally, we compared the
$ W{'} $ model and scalar LQ model together with the experiment and the SM in$ 2 $ D ($ \mathcal{B}({D}_{\left(s\right)}^{+}\to \eta {l}^{+}{\nu }_{l}) $ ,$ \mathcal{B}({D}_{\left(s\right)}^{+}\to {\eta }{{'}}{l}^{+}{\nu }_{l}) $ ) plane. These comparisons are shown in Fig 9. For$ {D}_{s}^{+}\to {\eta }{\left({'}\right)}{\mu }^{+}{\nu }_{\mu } $ decays (Fig. 9(a)), the branching fraction results from the scalar LQ model are substantially closer to the experimental results. The results using$ {W}' $ model are found within the SM and experimental values. Moreover, our results are found to be more consistent with the recent results determined by the BESIII collaboration [25]. In$ {D}_{s}^{+}\to {\eta }^{\left({'}\right)}{e}^{+}{\nu }_{e} $ decay (Fig. 9(b)), the values in LQ model have been observed to shift toward the experimental results. Using$ {W}' $ model, we observed slight deviation from the SM for these decay channels. To date,$ {D}^{+}\to {\eta }{{'}}{\mu }^{+}{\nu }_{\mu } $ decay is not examined experimentally. Hence, we compared our NP results with the SM results for$ {D}^{+}\to {\eta }^{\left({'}\right)}{\mu }^{+}{\nu }_{\mu } $ decays (Fig. 9(c)), and same deviation was obtained in both models. For$ {D}^{+}\to {\eta }^{\left({'}\right)}{e}^{+}{\nu }_{e} $ decays (Fig. 9(d)), we observed significant deviation compared to the$ {W}' $ model in scalar LQ model. Based on this comparative study, it is clear that the scalar LQ model is more reliable than the$ {W'} $ model for examining$ c\to (s,d)\overline{l}{\nu }_{l} $ transitions. -
Although recent experimental results on purely leptonic and semileptonic decays of
$ D $ mesons coincide with the theoretical predictions, a clear tension between theory and experiment has been observed on${D}_{\left(s\right)}^{+}\to {\eta }^{\left({'}\right)}{l}^{+}{\nu }_{l}$ decays. Hence, some NP may exist in these channels. In this study, we investigated${D}_{\left(s\right)}^{+}\to {\eta }^{\left({'}\right)}{l}^{+}{\nu }_{l}$ decays in$ {W}' $ model and scalar LQ model to obtain potential NP footprint. Furthermore, we tried to explain the experimental results of branching fractions associated to these decays using these two NP models. In Ref. [23], these decay modes have been examined with$ S U\left(3\right) $ flavour symmetry approach. Changing the hadronic form factors, they constructed four different cases by considering$S U\left(3\right)$ flavour symmetry and symmetry breaking. In certain cases, the predicted branching fraction results are within$ 2\sigma $ error range of the experimental results and some results are also consistent with experimental data. In the Refs. [18, 19], these channels have been investigated in a model independent manner. The NP contributions were directly considered from the four-fermion operators (such as vector, scalar, and tensor operators) individually, and the deviations from the SM have been shown graphically. In the current study, we considered the contributions of the aforementioned NP operators via the incorporations of new coupling parameters of the new particles such as$ {W}' $ boson and leptoquark. To structure these NP models, the coupling parameters are predicted from the recent experimental branching fraction results of semileptonic leptonic, and$ D $ meson decays. Different decay observables, such as branching fraction, forward-backward asymmetry, and lepton polarization asymmetry are examined within these NP models. Our predicted values of branching fractions in$ {W}' $ model approach toward the experimental results and are approximately in the$ 1.5\sigma $ region. This model is unable to produce NP signal on forward-backward asymmetry and lepton polarization asymmetry because the NP dependent vector Wilson coefficient$ {C}_{{V}_{L}} $ neutralizes these observables. Hence,$ {W}'$ model is solely suitable for examining branching fraction. Instead of considering the four-fermion operators individually (as done in Refs. [18, 19]), we considered the combined impact of all these operators from their corresponding vector ($ {C}_{{V}_{L}} $ ), scalar ($ {C}_{{S}_{L}} $ ), and tensor ($ {C}_{T} $ ) Wilson coefficients within the context of a scalar LQ model. Our predicted branching fraction results are approximately in the$ 1\sigma $ range of the experimental results, excluding$ {D}_{s}^{+}\to \eta {e}^{+}{\nu }_{e} $ and${D}^{+}\to {\eta }{{'}}{e}^{+}{\nu }_{e}$ decays. Furthermore, the effectiveness of our models is clearly observed through the comparison of our predicted results with the experimental results and SM predictions in 2D branching fraction plane. The key feature of the scalar LQ model is its capability of combining the impact of these operators together. Furthermore, given the extremely small values of$ {C}_{T} $ , we did not observe any effect of scalar LQ on forward-backward asymmetry and lepton polarization asymmetry. Thus, based on the aforementioned analysis, it is clear that the scalar LQ model is more reliable for examining$ c\to (s,d)\overline{l}{\nu }_{l} $ transitions in the framework of NP. Specifically, our predicted values in scalar LQ model,$\mathcal{B}\left({D}_{s}^{+}\to \eta {\mu }^{+}{\nu }_{\mu }\right) =(1.73\pm 0.41)\times {10}^{-2}$ and$\mathcal{B}\left({D}_{s}^{+}\to {\eta }{{'}}{\mu }^{+}{\nu }_{\mu }\right)=(6.42\pm 1.47)\times {10}^{-3}$ , are consistent with the recent results by the BESIII collaboration [25],$(2.215\pm 0.051\pm 0.052)\times {10}^{-2}$ and$\left(8.01\pm 0.55 \pm 0.31\right)\times {10}^{-3}$ , respectively. The allowed coupling parametric space of the NP models, with the mass of these heavy bosons at$ ~1 $ TeV, reduces the deviation from the current experimental constraints (as shown in Table 7). We will be able to better comprehend the existence of NP with the aid of impending measurement of$ D $ meson decays in the BESIII and Belle II experiments. We expect that our predicted values in scalar LQ model will be closer to the outcomes of upcoming experiments. -
Parameter Value $ {G}_{F} $ 

$ 1.1663787\times {10}^{-5} $ GeV−2

$ {V}_{cs} $ 

$ 0.987\pm 0.011 $ 

$ {V}_{cd} $ 

$ 0.221\pm 0.004 $ 

$ {m}_{D} $ 

$ 1869.66\pm 0.05 $ MeV

$ {m}_{{D}_{s}} $ 

$ 1968.35\pm 0.07 $ MeV

$ {\tau }_{D} $ 

$ \left(1.033\pm 0.005\right)\times {10}^{-12} $ s

$ {\tau }_{{D}_{s}} $ 

$ (5.04\pm 0.04)\times {10}^{-13} $ s

$ {m}_{\eta } $ 

$ 547.862\pm 0.017 $ MeV

$ {m}_{{\eta }^{{'}}} $ 

$ 957.78\pm 0.06 $ MeV

$ {m}_{\mu } $ 

$ 0.1056583745 $ GeV

$ {m}_{e} $ 

$ 0.51099895000 $ MeV

$ {m}_{c} $ 

$ 1.27\pm 0.02 $ GeV

$ {m}_{s} $ 

$ {93.4}_{-3.4}^{+8.6} $ MeV

$ {m}_{d} $ 

$ {4.67}_{-0.17}^{+0.48} $ MeV

Table A1. Values of the input parameters [24].
Model dependent analysis of $ {\boldsymbol D_{(\boldsymbol s)}^{\bf +} \to \boldsymbol\eta^{(')}\boldsymbol l^{\bf +}{\boldsymbol v}_{\boldsymbol l}}$ decays in beyond standard model
- Received Date: 2024-04-01
- Available Online: 2024-09-15
Abstract: Motivated by the recent experimental results of branching fractions for







 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF






















 DownLoad:
DownLoad: