-
While hyperons, i.e., baryons with a strangeness content, play an important role in compact star mergers and core-collapse events [1], there are limited experimental data on doubly strange hypernuclear systems, and the systems containing higher strangeness are almost unknown. Different phenomenological models have been developed for the nucleon-hyperon (NY) and hyperon-hyperon (YY) interactions. Nonetheless, recent developments in computational technologies and theoretical progress in Lattice QCD methods facilitated the derivation of
\Omega N ,\Omega \Omega ,\Lambda \Lambda , andN \Xi interactions [2–4], close to the physical pion massesm_{\pi} \simeq 146 MeV and Kaon massesm_k\simeq525 MeV, by the HAL QCD Collaboration [5, 6], where their physical values arem_{\pi} \simeq 135 MeV andm_k\simeq497 MeV. TheN\Omega and di-\Omega interactions were suggested and predicted before the lattice QCD simulation in [7, 8].The potentials are obtained on a large space-time volume
L^{4}=(8.1\ {\rm{fm}})^{4} with a lattice spacinga=0.0846 fm. While there are sophisticated calculations to study{}_{\Lambda\Lambda }^{\;\;\;6}{{\rm{He}}} hypernucleus [9–18], in this work, we examine the HAL QCD\Lambda \Lambda interactions, which are the most consistent potential with the LHC ALICE data [19, 20], to study the ground state properties of the{}_{\Lambda\Lambda }^{\;\;\;6}{{\rm{He}}} hypernucleus. Similarly, we explore the\Omega \Omega \alpha system with lattice QCD-based interactions.The following motivated our exploration for possible implications of the attractive nature of the
\Omega N and\Omega \Omega interactions on few-body\Omega \Omega \alpha systems on the basis of first-principle lattice QCD-based interactions. In few-body systems the presence of additional nucleons may increase the binding, as demonstrated in many straightforward examples in nature. Although there are no dibaryon bound states with strangeness=-1 (\Lambda -nucleon system), hypertriton_{\Lambda}^{3} H, consisting of a neutron, a proton, and a\Lambda -particle, is bound with a separation energy of0.41 \pm 0.12 MeV [21, 22]. In the case of strangeness=-2 , in systems containing\Xi -particles, an enhancement in the binding energy per baryon is observed by increasing the number of nucleons [23–25]. The Extended-Soft-Core (ESC08c) model of Nijmegen interaction [26] supports the bound states of\Xi N and\Xi N N \ (T=1/2, \ J^{\pi} = 3/2^+) with energies-1.56 and-17.2 MeV, respectively [23, 24, 27]. Recently Garcilazo et al. have implemented\Omega N and\Omega \Omega interactions derived by the HAL QCD Collaboration [2, 3, 28] to study three-body systems containing more strangeness number, i.e.,\Omega N N and\Omega \Omega N with strangeness= -3 and-6 [26]. As a result, they obtained\Omega d \ (0, \ 5/2^+) binding energy of about-20 MeV and two resonance states\Omega nn \ (1, \ 3/2^+) and\Omega \Omega N \ (1/2, \ 1/2^+) , with resonance energies of1 and4.6 MeV, correspondingly. Besides the\Omega -deuteron bound state, the\Omega \Omega \alpha bound state would be an interesting system to benchmark the\Omega \alpha and\Omega \Omega interactions in a three-body system.As the femtoscopic analysis of two-particle correlation functions in heavy-ion collisions provides information on hadron-hadron interactions at low energies [29, 30], investigating the
{}_{\Omega\Omega }^{\;\;\;6}{{\rm{He}}} system can also be interesting for this purpose. The correlation function in multistrange systems such as\Lambda \Lambda [31, 32] andp \Omega [20, 33, 34] have been measured recently in high-energy nuclear collisions. Furthermore, as a next step in femtoscopic analyses, the hadron-deuteron correlation functions would be promising. So far, experimental investigations of correlations for pd, dd and even for light nuclei have been already performed [35–37], whereas theK^{-}d case is currently in progress [38, 39], and\Lambda d correlation function is in the pipeline [40, 41]. A method to probe the momentum correlation functions of\Omega \Omega is proposed in Ref. [42]. Very recently, the production of\Omega NN and\Omega \Omega N in ultra-relativistic heavy-ion collisions using the Lattice QCD\Omega N ,\Omega \Omega potentials has been studied in Ref. [43]. Since the di-Omega appears with the binding energy approximately1.6 MeV in^1S_{0} channel [2], there is a possibility that our results could help the future study of\Omega \Omega -\alpha (liked-\alpha [44]) two-particle momentum correlation functions, and can be measured in high energy heavy-ion collisions. We explore this hypothetical system for the first time, and to the best of our knowledge, there is no other study performed on this system thus far.In the present work, we study the ground state properties of
{}_{\Lambda\Lambda }^{\;\;\;6}{{\rm{He}}} and{}_{\Omega\Omega }^{\;\;\;6}{{\rm{He}}} hypernuclei as a three-body(\alpha + Y + Y) bound state. For this, we solve two coupled Faddeev equations in configuration space with the hyperspherical harmonics expansion method to calculate the ground state binding energy and the geometrical structures of these hypernuclei. In our study, we use the HAL QCD\Lambda \Lambda and\Omega \Omega interactions, Isle-type Gaussian potential for\alpha \Lambda interactions and a Woods-Saxon type potential for\alpha \Omega interaction.In 2001, the KEK emulsion/scintillating-fiber hybrid experiment [45], known as the Nagara event, reported a uniquely identified double-
\Lambda hypernucleus{}_{\Lambda\Lambda }^{\;\;\;6}{{\rm{He}}} with binding energy of-7.25\pm0.19 MeV. The re-analysis of the Nagara event using the new\Xi mass of1321.71 MeV [46], revised the binding energy to-6.91\pm0.16 MeV [47, 48], considerably shallower than the earlier measured value-10.9\pm0.8 MeV [49]. In the recent J-PARC E07 experiment emulsion analysis, several hypernuclear events have been observed. For example, theBe double\Lambda hypernucleus has been identified as an event called the “MINO event” [50], and a new\Xi -nuclear deeply bound state has been reported [51]. Furthermore, the high precision spectra for light to heavy multi-strange hypernuclei are planned to be measured in the future at JLab and with the new high-intensity high-resolution line at [51, 52].Hiyama et al. performed a three-body calculation for
\Lambda+\Lambda+{}_{ }^4{{\rm{He}}} , with the Gaussian expansion method, using properly tuned\Lambda \Lambda Nijmegen interactions to reproduce the Nagara event data [9, 10]. Nemura et al. studied\Lambda \Lambda hypernuclei with the stochastic variational method using effective phenomenological\Lambda N and\Lambda\Lambda potentials [11, 12]. Moreover, Filikhin et al. have studied{}_{\Lambda\Lambda }^{\;\;\;6}{{\rm{He}}} by solving the differential Faddeev equations (DFE) in configuration space using different models of NijmegenYY interactions [13–16]. Recently, double-strangeness hypernuclei were studied in an effective field theory approach using the stochastic variational method at leading order [17] and with the Jacobi no-core shell model at the next-to-leading order [18]. The cluster structure of light hypernuclei [53, 54] has been studied with different methods, including the generator coordinate method [55], orthogonality condition model [56], Gaussian expansion method [57, 58], and Tohsaki-Horiuchi-Schuck-Röpke wave function approach [59].The Faddeev-Yakubovsky (FY) equations are extensively used to study the structure of three- and four-body bound states, with identical and non-identical particles, in different sectors of physics [60–65]. FY equations are solved with different techniques such as direct projection in momentum space [66], hyperspherical harmonics (HH) [67], adiabatic hyperspherical [68], and variational methods [69, 70]. The HH method has been implemented to study the complex structure of
{\rm{{^{6}}}He} and{\rm{{^{11}}}Li} halo nuclei in a three-body picture [67, 71]. In this work, we apply this method to study the ground state properties of double hyperon nuclei in a three-body picture asY+Y+\alpha .In Sec. II, we briefly review the HH formalism for
Y+Y+\alpha three-body bound state. In Sec. III, we introduceYY and\alpha Y two-body potentials used to study the structure of double-hyperon nuclei. Our numerical results for the ground state binding energies and geometrical properties of{}_{\Lambda\Lambda }^{\;\;\;6}{{\rm{He}}} and{}_{\Omega\Omega }^{\;\;\;6}{{\rm{He}}} hypernuclei are presented and discussed in Sec. IV. A summary and outlook is provided in Sec. V. -
The total wave function
\Psi^{j \mu} of the three-body system(YY \alpha) for a given total angular momentumj by projection\mu , composed of twoY particles and one\alpha particle, is given as a sum of three Faddeev components\psi_i^{j \mu} \Psi^{j \mu}=\sum\limits_{i=1}^{3}\psi_{i}^{j \mu}\left({\boldsymbol{x}}_{i}, {\boldsymbol{y}}_{i}\right).
(1) Each component
\psi_i^{j \mu} is expressed in terms of two Jacobi coordinates({\boldsymbol{x}}_{i}, {\boldsymbol{y}}_{i}) and can be obtained from the solution of coupled Faddeev equations\begin{array}{l} \left(H_0 - E\right)\psi_{i}^{j \mu}+V_{jk}\left(\psi_{i}^{j \mu}+\psi_{j}^{j \mu}+\psi_{k}^{j \mu}\right)=0, \end{array}
(2) where
H_0 is the free Hamiltonian,E is 3B binding energy, andV_{jk} is the two body interaction (both the Coulomb and nuclear interactions) between the corresponding pair. The indexesi, j, k run through\left(1, 2, 3\right) in circular order. To solve the coupled Faddeev equations (2) in configuration space, one needs two different sets of Jacobi coordinates( {\boldsymbol x}_1, {\boldsymbol y}_1) and( {\boldsymbol x}_3, {\boldsymbol y}_3) , as shown in Fig. 1, defined by
Figure 1. (color online) Two sets of Jacobi coordinates
(Y\alpha, Y) and(YY, \alpha) for a description of theYY \alpha three-body system.\begin{aligned}[b] {\boldsymbol x}_i =& \sqrt{A_{jk}} \ {\boldsymbol r}_{jk} = \sqrt{A_{jk}} \ ( {\boldsymbol r}_j - {\boldsymbol r}_k) , \\ {\boldsymbol y}_i =& \sqrt{A_{(jk)i}} \ {\boldsymbol r}_{(jk)i} = \sqrt{A_{(jk)i}} \left( {\boldsymbol r}_i - \frac{A_j {\boldsymbol r}_j + A_k {\boldsymbol r}_k}{A_j+A_k} \right) , \end{aligned}
(3) where
{\boldsymbol r}_i is the position vector of particlei ,{\boldsymbol r}_{jk} is the relative distance between the pair particles(jk) , and{\boldsymbol r}_{(jk)i} is the distance between the spectator particlei and the center of mass of pair(jk) . The reduced masses areA_{jk}=\dfrac{A_jA_k}{A_j+A_k} andA_{(jk)i}=\dfrac{(A_j+A_k)A_i}{A_i+A_j+A_k} , whereA_i=\dfrac{m_i}{m} ,m=1 a.m.u., andm_i is the mass of particlei in a.m.u.The projection of coupled Faddeev equations onto the Jacobi coordinates
( {\boldsymbol x}_i , {\boldsymbol y}_i ) leads to two-dimensional partial differential equations that can be transformed into two sets of coupled one-dimensional equations using the hyperspherical coordinates( \rho_i, \Omega_i ) . The hyperradius is defined by\rho_i^{2}=x_i^{2}+y_i^{2} ; the angular part\Omega_i \equiv \{ \theta_i, \hat{ {\boldsymbol x}}_i, \hat{ {\boldsymbol y}}_i \} denotes a set of hyperspherical angles, with the hyperangle\theta_i=\arctan (x_i/y_i) and other angles associated with the unit vectors\hat{ {\boldsymbol x}}_i and\hat{ {\boldsymbol y}}_i . The projection of Faddeev components\psi_{Y} and\psi_{\alpha} , hereafter shown as\psi_{ Q} , for a given total angular momentumj and its projection\mu onto the spherical coordinates is given by [72, 73],\psi_{ Q}^{j \mu} (\rho_i, \Omega_i ) = \rho_i^{-5/2} \sum\limits_{\beta}\mathcal{R}_{\beta}^{j} (\rho_i )\mathcal{Y}_{\beta}^{j \mu} (\Omega_i),
(4) \mathcal{Y}_{\beta}^{j\mu}\left(\Omega_{i}\right) is written in terms of hyperspherical harmonics\Upsilon_{Kl}^{l_{x_{i}}l_{y_{i}}}\left(\Omega_{i}\right) , which are eigenstates of the hypermomentum harmonics operator\hat{K} as\begin{array}{l} \mathcal{Y}_{\beta}^{j\mu}\left(\Omega\right)=\left\{ \left[\Upsilon_{Kl}^{l_{x}l_{y}}\left(\Omega\right)\otimes\phi_{S_{x}}\right]_{j_{ab}}\otimes\kappa_{I}\right\} _{j\mu}, \end{array}
(5) \begin{array}{l} \Upsilon_{Klm_{l}}^{l_{x}l_{y}}\left(\Omega \right)=\varphi_{K}^{l_{x}l_{y}}\left(\theta \right)\left[Y_{l_{x}}\left(\hat{x}\right)\otimes Y_{l_{y}}\left(\hat{y}\right)\right]_{lm_{l}}, \end{array}
(6) \begin{array}{l} \varphi_{K}^{l_{x}l_{y}}\left(\theta\right)=N_{K}^{l_{x}l_{y}}\left(\sin\theta\right)^{l_{x}}\left(\cos\theta\right)^{l_{y}}P_{n}^{l_{x}+1/2, l_{y}+1/2}\left(\cos2\theta\right), \end{array}
(7) where
P_{n}^{a, b} is the Jacobi polynomial of ordern=\left(K-l_{x}-l_{y}\right)/2 , andN_{K}^{l_{x}l_{y}} is a normalizing coefficient. The parameter\beta \equiv \{ K, l_{x}, l_{y}, l, S_{x}, j_{ab}\} represents a set of quantum numbers of a specific channel coupled toj .K is the hyperangular quantum number,l_{x} andl_{y} are the orbital angular momenta of the Jacobi coordinatesx andy ,{\boldsymbol{l}}={\boldsymbol{l}}_{x}+{\boldsymbol{l}}_{y} is total orbital angular momentum,S_{x} is the total spin of the pair particles associated with the coordinatex , and{\boldsymbol{j}}_{ab}={\boldsymbol{l}}+{\boldsymbol{S}}_{x} .{\boldsymbol{I}} denotes the spin of the third particle, and the total angular momentumj is{\boldsymbol{j}}={\boldsymbol{j}}_{ab}+{\boldsymbol{I}} . In Eq. (5),\phi_{S_{x}} is the spin wave function of two-body subsystem, and\kappa_{I} is the spin function of the third particle. Applying this expansion in the Faddeev equations and performing the hyperangular integration, one obtains a set of coupled differential equations for the wave functions\mathcal{R}_{\beta}^{j}\left(\rho\right) of Eq. (4) as\begin{aligned}[b]& \left[-\frac{\hbar^{2}}{2m}\left(\frac{{\rm d}^{2}}{{\rm d}\rho^{2}}-\frac{\left(K+3/2\right)\left(K+5/2\right)}{\rho^{2}}\right)-E\right]\mathcal{R}_{\beta}^{j}\left(\rho\right) \\& +\mathop{\sum\limits_{\beta^{\prime}}}V_{\beta\beta^{\prime}}^{j\mu}\left(\rho\right)\mathcal{R}_{\beta^{\prime}}^{j}\left(\rho\right)=0, \end{aligned}
(8) The coupling potentials are the hyperangular integrations of the two-body interaction
V_{\beta\beta^{\prime}}^{j\mu}\left(\rho_{i}\right)= \langle \mathcal{Y}_{\beta}^{j\mu}\left(\Omega_{i}\right) \left| {\hat{V}_{ij}} \right|\mathcal{Y}_{\beta^{\prime}}^{j\mu}\left(\Omega_{j}\right)\rangle , and\hat{V}_{ij} are the two-body potentials between particlesi andj , which will be introduced in Section III.In order to solve these coupled equations, the hyperradial wave functions
\mathcal{R}_{\beta}^{j} (\rho) are expanded in a finite basis set ofi_{\max} hyperradial excitations as\mathcal{R}_{\beta}^{j} (\rho)=\sum\limits_{i=0}^{i_{\max}}C_{i\beta}^{j}R_{i\beta}(\rho),
(9) where the coefficients
C_{i\beta}^{j} can be obtained by diagonalizing the three-body Hamiltonian fori=0, ..., i_{\max} basis functions. The hyperradial functionsR_{i\beta}(\rho) can be written in terms of Laguerre polynomials.By having the three-body wave function
\Psi^{j \mu} in the hyperspherical coordinates, one can study the geometrical structure ofYY \alpha systems by calculating the matter radiusr_{\rm mat}=\sqrt{\langle r^{2}\rangle } and the correlation densities, giving the probability to have definite distances between the particles in the three-body system:P(r_{jk}, r_{(jk)i}) = \frac{r^2_{jk} r^2_{(jk)i}}{2j+1} \sum\limits_{\mu} \int {\rm d} \hat{x}_{i} {\rm d}\hat{y}_{i} \left| {\psi^{j \mu} (x_i, y_i)} \right|^2.
(10) -
In this section, we present the two-body interactions, which we use in our calculations for the bound state of
\Lambda\Lambda\alpha and\Omega\Omega\alpha three-body systems. -
For
\Lambda \Lambda interaction, we use HAL QCD potentials in^{1}S_{0} channel with isospinT=0 [4]\begin{aligned}[b] V_{\Lambda\Lambda}(r)=& \sum\limits_{i=1}^{2} \alpha_{i}\exp(-r^{2}/\beta_{i}^{2}) \\ &+ \lambda_{2} \left (1-\exp(-r^{2}/\rho_{2}^{2}) \right)^{2} \left( \frac{\exp(-m_{\pi}r)}{r} \right)^{2}. \end{aligned}
(11) The effective
\Lambda \Lambda interaction is handled by the coupled-channel formalism [74] at three imaginary-time distances oft/a=11, 12, 13 , where the potential parameters are fitted to\chi^{2}/d.o.f = 1.30(40), \ 0.76(18) , and0.74(30) , respectively. Thet -dependence is insignificant within the statistical errors. The fitted potential parameters to Eq. (11) are given in Table 1, where the pion mass ism_{\pi}=146 MeV.t/a 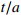
\alpha_{1} / {\rm{MeV} } 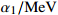
\beta_{1} / { {\rm{fm} } } 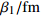
\alpha_{2} / {\rm{MeV} } 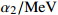
\beta_{2} / {\rm{fm} } 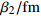
\lambda_{2} / {\rm{MeV \cdot fm} }^{2} 
\rho_{2} / {\rm{fm} } 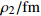
11 1466.4 0.160 407.1 0.366 −170.3 0.918 12 1486.7 0.156 418.2 0.367 −160.0 0.929 13 1338.0 0.143 560.7 0.322 −176.2 1.033 Table 1. Fitted parameters of
V_{\Lambda \Lambda}(r) potential, shown in Eq. (11), taken from Ref. [4]. The statistical errors in fitted parameters are not taken into account in our calculations.The low-energy data derived from this interaction indicate no bound or resonant di-hyperon around the
\Lambda\Lambda threshold in (2+1)-flavor QCD at nearly physical quark masses. These data predict a scattering lengtha_{0}^{\left(\Lambda\Lambda\right)}=-0.81\pm0.23_{-0.13}^{+0.00} fm and an effective ranger_{ {\rm{eff}}}^{\left(\Lambda\Lambda\right)}=5.47\pm0.78_{-0.55}^{+0.09} fm. The central values and the statistical errors are extracted from phase shifts att/a=12 , whereas the systematic errors are estimated from the central values att/a=11 and13 [4]. The systematic errors are estimated by the difference between the results obtained by the fit range, and the statistical errors are estimated by the jackknife sampling of the lattice QCD configurations. The source of systematic error is the contamination from inelastic states.For the
\Lambda \alpha interaction we use the Isle-type Gaussian potential [14]\begin{array}{l} V_{\Lambda\alpha}(r)=450.4 \exp(-(r/1.25)^{2})-404.9 \exp(-(r/1.41)^{2}). \end{array}
(12) This potential reproduces the experimental data for the lifetime and binding energy of the
_{\Lambda}^{5}\rm He hypernucleus with\tau = 3.02_{-0.09}^{+0.10}\times10^{-10} s andE_{B} = -3.1 MeV [75]. -
The HAL QCD
\Omega \Omega potential in the^{1}S_{0} channel is fitted to an analytical function as [2]V_{\Omega\Omega}(r)=\sum\limits_{j=1}^{3}c_j {\rm e}^{-(r/d_j)^{2}},
(13) where the potential parameters, without considering the statistical errors, are
(c_{1}, \ c_{2}, \ c_{3}) = (914, \ 305, \ -112) MeV and(d_{1}, \ d_{2}, \ d_{3}) = (0.143, \ 0.305, \ 0.949) fm. Using a single-folding potential method, an\Omega\alpha interaction has been recently extracted from a separable HAL QCD\Omega N potential. This potential supports an\Omega\alpha bound state with a binding energy of approximately-22 MeV and is parameterized in the form of the Woods-Saxon type potential [76]V_{\Omega\alpha}(r)=-61 \left (1+ \exp \left (\frac{r-1.7}{0.47} \right) \right) ^{-1}.
(14) All two-body interactions for
YY (\Lambda\Lambda and\Omega\Omega ) and alsoY\alpha (\Lambda\alpha and\Omega\alpha ) subsystems are shown in Fig. 2.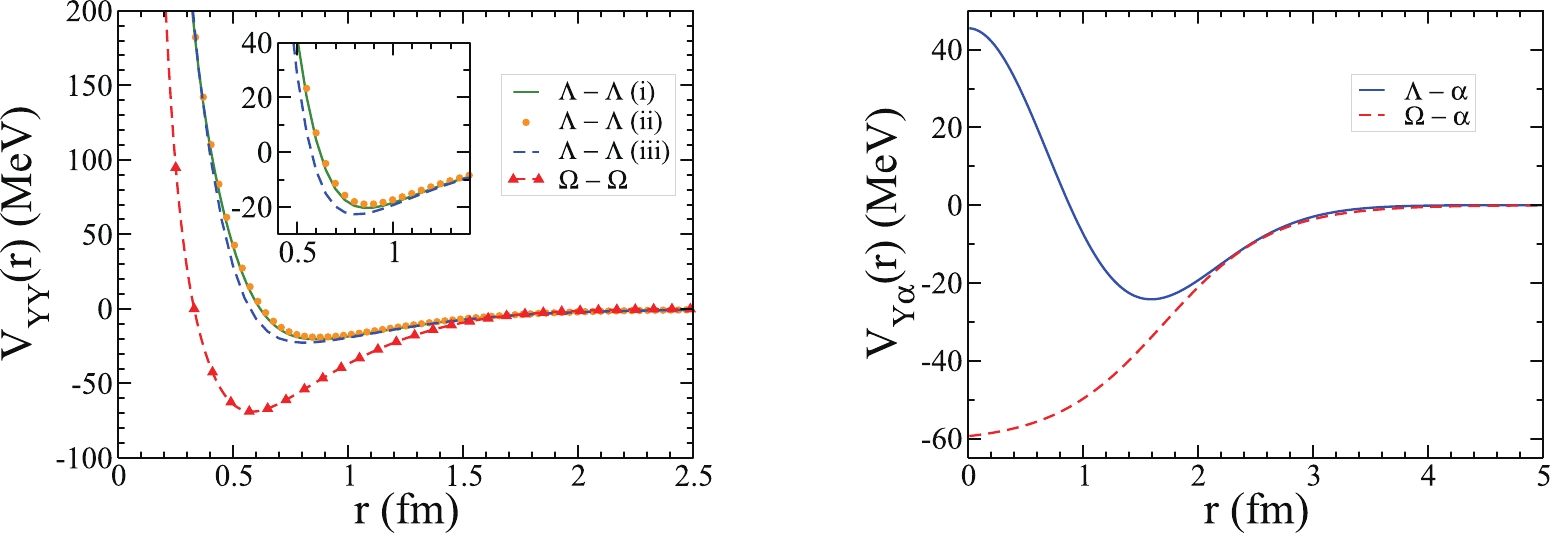
Figure 2. (color online) Left panel: YY potentials for three models of the HAL QCD
\Lambda\Lambda potential of Eq. (11) with the parameters given in Table 1 at the imaginary-time distancest/a=11, 12, 13 (shown as model i, ii, iii) and the HAL QCD\Omega\Omega potential of Eq. (13). Right panel:Y\alpha potentials for the Isle-type\Lambda\alpha potential given in Eq. (12) and the Woods-Saxon type\Omega\alpha potential given in Eq. (14).In our calculations, we consider the Coulomb interaction in the
\Omega\Omega\alpha system using a hard-sphere model as [77]\begin{array}{ll} V_{\rm Coul}\left(r\right)=Z^{2}e^{2}\times\begin{cases} \dfrac{1}{r_{\rm Coul}}\left(\dfrac{3}{2}-\dfrac{r^{2}}{2r_{\rm Coul}^{2}}\right), & r\leq r_{\rm Coul}\\ \dfrac{1}{r}, & r > r_{\rm Coul} \end{cases} \end{array}
(15) with a Coulomb radius
r_{\rm Coul} = 1.47 fm. -
To calculate the ground state binding energy and the geometrical properties of
\Lambda \Lambda \alpha and\Omega \Omega \alpha , we solve the coupled Faddeev equations (2) by implementing the FaCE computational toolkit [78] using the two-body interactions discussed in Sec. III. To discretize the continuous hyperradius coordinate\rho_i , we use the Gauss-Laguerre quadrature with 100 grid points, while the hyperangular integrations are performed using the Gauss-Jacobi quadrature with 60 grid points. The hyperradius cutoffs are selected high enough to achieve the cutoff-independent binding energies, converging with four significant figures. In our calculations, the masses of particles arem_{N} = 939 MeV,m_{\Lambda} = 1127.42 MeV,m_{\Omega}=1672.45 , andm_{\alpha} = 3727.38 MeV. Table 2 shows the convergence of the three-body ground state binding energyE_{3} and nuclear matter radiusr_{\rm mat} as a function of the maximum values of hyperangular quantum numberK_{\max} and hyperradial excitationsi_{\max} . The spin and isospin of\Lambda\Lambda\alpha and\Omega\Omega\alpha systems are equal to zero. The number of strange quark content for\Lambda\Lambda\alpha and\Omega\Omega\alpha is equal to 2 and 6, respectively. The Coulomb interaction of Eq. (15) is considered in\Omega\Omega\alpha systems leading to an increase of approximately6 MeV in 3B binding energy.\Lambda\Lambda\alpha 
\Omega\Omega\alpha 
i_{\max} 
E_{3} /MeV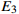
r_{\rm mat} /fm
E_{3} /MeV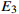
r_{\rm mat} /fm
5 −7.442 1.930 −67.19 1.327 10 −7.467 1.954 −67.21 1.326 15 −7.468 1.955 −67.21 1.326 20 −7.468 1.955 −67.21 1.326 25 −7.468 1.955 −67.21 1.326 K_{\max} 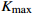
E_{3} /MeV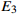
r_{\rm mat} /fm
E_{3} /MeV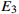
r_{\rm mat} /fm
5 −6.897 1.953 −66.58 1.327 10 −7.321 1.948 −67.04 1.326 15 −7.404 1.951 −67.14 1.326 20 −7.446 1.953 −67.19 1.326 25 −7.456 1.954 −67.20 1.326 30 −7.463 1.955 −67.21 1.326 35 −7.465 1.955 −67.21 1.326 40 −7.466 1.955 −67.21 1.326 45 −7.467 1.955 −67.21 1.326 50 −7.467 1.955 −67.21 1.326 55 −7.467 1.955 −67.21 1.326 60 −7.468 1.955 −67.21 1.326 65 −7.468 1.955 −67.21 1.326 70 −7.468 1.955 −67.21 1.326 75 −7.468 1.955 −67.21 1.326 80 −7.468 1.955 −67.21 1.326 Table 2. Convergence of three-body ground state binding energy
E_{3} and nuclear matter radiusr_{\rm mat} calculated for\Lambda\Lambda\alpha and\Omega\Omega\alpha systems as a function of maximum hyperradial excitationsi_{\max} (withK_{\max}=80 ) and hyperangular quantum numberK_{\max} (withi_{\max}=25 ).Since
\Omega \alpha interaction is completely attractive, faster convergence is reached in the calculation of ground state binding energy and nuclear matter radius of\Omega\Omega\alpha to-67.21 MeV and1.326 fm. For the\Lambda\Lambda\alpha system, a convergence can be reached at larger values ofK_{\max} andi_{\max} . For\Lambda\Lambda\alpha , the ground state binding energy and nuclear matter radius converge to-7.468 MeV and1.955 fm. The listed results for\Lambda\Lambda\alpha are obtained for the\Lambda\Lambda interaction with imaginary-time distancet/a=12 , whereas fort/a=11 andt/a=13 , the calculated ground state binding energies are-7.605 MeV and-7.804 MeV, respectively.In Table 3, in addition to the converged 3B binding energies for
\Lambda\Lambda\alpha and\Omega\Omega\alpha systems, we list the binding energy of two-bodyYY andY \alpha subsystems. Furthermore, the 3B binding energies in which the 2B interactions between identical hyperons are set to zero, i.e.,V_{YY}=0 , are listed. Our numerical results show that the relative percentage difference(B_3 (V_{YY}=0) - 2B_2(V_{Y\alpha}) ) / B_3 (V_{YY}= 0) \times 100 varies between4 to 6%. As one can see in Table 3, the\Omega\Omega\alpha has a deeper bound state by having two bound subsystems. Our numerical analysis shows that the uncertainties in the HAL QCD\Omega\Omega (\Lambda \Lambda ) potential parameters impact the{}_{\Omega\Omega }^{\;\;\;6}{{\rm{He}}} ({}_{\Lambda\Lambda }^{\;\;\;6}{{\rm{He}}} ) ground state binding energy for approximately4 (0.1 ) MeV and ther_{\rm mat} for less than0.01 (0.007 ) fm. While the employed\Omega \alpha potential in our calculations is derived based on the dominant^{5}S_{2} channel ofN \Omega interactions [3, 79], the contribution of the^{3}S_{1} channel can be reasonably ignored. Although the implemented two-body interactions in our calculations are restricted to only one angular momentum channel, we should point out that, to the best of our knowledge, no lattice two-body interactions developed to higher channels. This restriction in the interactions should explain the deep binding of the\Omega particles to the\alpha as possible contributions of repulsive channels, which are not taken into account, even though their contributions appear to be small [79].YY \alpha System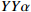
E_{2}\; (YY ) 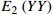
E_{2}\; (Y \alpha) 
E_{3} 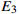
E_{3} (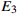
V_{YY }=0 )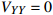
\Lambda\Lambda\alpha \; (t/a=12) 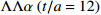
Not Bound -3.146 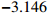
-7.468 
-6.463 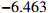
\Omega\Omega\alpha 
-1.408 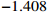
-22.01 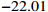
-67.21 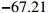
-48.96 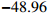
Table 3. Three-body ground state binding energies
E_{3} in MeV forYY \alpha systems. The last column shows our results for 3B binding energies with zero interaction inYY subsystems. Two-body binding energiesE_{2} forYY andY \alpha subsystems are also shown in MeV.In Table 4, we compare our numerical results with other theoretical results such as those of the Gaussian expansion method (GEM), stochastic variational method (SVM), differential Faddeev equations (DFE), quark-cluster-model (QCM) by different
YY interaction models like the Nijmegen model D (ND), simulating Nijmegen hard-core model F(NF_{s}) , modified simulating Nijmegen hard-core model D(mND_{s}) , simulating Nijmegen hard-core model D(ND_{s}) , spin-flavorSU6 quark-model (fss2), Nijmegen soft-core model (NSC97e), G-matrix interaction based on the bare ND interaction (ND(G)), and Nijmegen extended soft-Core (ESC00) model as well as the experimental data (Exp).Ref. YY Model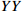
E_{3} /MeV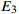
present HAL QCD (t/a=11) 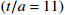
-7.605 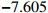
present HAL QCD (t/a=12) 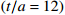
-7.468 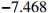
present HAL QCD (t/a=13) 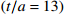
-7.804 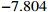
[10] (GEM) ND -7.25 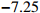
[12] (SVM) NF_{s} 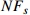
-7.52 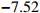
[12] (SVM) mND_{s} 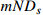
-7.53 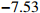
[12] (SVM) ND_{s} 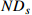
-7.93 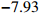
[80] (DFE) fss2 -7.653 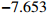
[14] (DFE) NSC97e -6.82 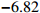
[14] (DFE) ND -9.10 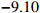
[14] (DFE) ND(G) -10.1 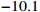
[14] (DFE) ESC00 -10.7 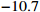
[81] (QCM) ND -9.7 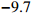
[81] (QCM) ND(G) -9.4 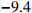
[82] (GEM) ND -9.34 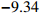
[83](G-matrix) ND(G) -9.23 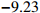
[47, 48] (Exp.) – -6.91 \pm 0.16 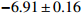
[45] (Exp.) – -7.25\pm0.19 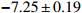
[49] (Exp.) – -10.9\pm0.8 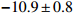
Table 4. Comparison between our results for three-body ground state binding energy
E_{3} of\Lambda\Lambda\alpha system and other theoretical and experimental data.By having 3B wave functions of
YY \alpha systems in terms of the HH basis, we calculate the geometrical quantities, i.e., the r.m.s. distances between the particles and the r.m.s. matter radius, presented in Table 5. In our calculations, we consider the\alpha matter radius of1.47 fm. For comparison, we also present the DFE results in Ref. [14]. Since the studied 3B systems consist of two identical hyperons and one alpha particle, interacting by scalar potentials, three particles form a ground state, where the most probable positions of the particles have the shape of an isosceles triangle. As shown in Table 5, our numerical results for the expectation values of the Jacobi coordinates in(YY, \alpha) and(Y\alpha, Y) configurations satisfy the following Pythagorean theorem with high accuracy(YY \alpha) system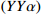
\langle r_{YY} \rangle /fm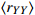
\langle r_{Y \alpha} \rangle /fm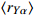
\langle r_{(YY )\alpha} \rangle /fm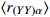
\left| {\Delta} \right| /fm
^2 
\langle \rho^{2}\rangle^{1/2} /fm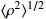
r_{\rm mat} /fm
\Lambda\Lambda\alpha\; (t/a=11) 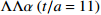
3.598 (3.36) 2.902 (2.70) 2.276 (2.11) 0.005 (0.015) 3.943 1.944 \Lambda\Lambda\alpha\; (t/a=12) 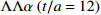
3.629 2.926 2.295 0.002 3.976 1.955 \Lambda\Lambda\alpha\; (t/a=13) 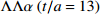
3.555 2.867 2.248 0.007 3.895 1.929 \Omega\Omega\alpha 
1.521 1.293 1.047 0.003 2.037 1.326 Table 5. Expectation values of Jacobi coordinates in
\Lambda\Lambda\alpha and\Omega\Omega\alpha systems.\langle r_{YY} \rangle is the separation between identical hyperons,\langle r_{Y\alpha} \rangle is the separation betweenY\alpha pairs, and\langle r_{(YY)\alpha} \rangle is the separation between the center of mass ofYY pair and the spectator\alpha particle.\langle \rho^{2}\rangle^{1/2} is the r.m.s. matter radius of the three-body system containing only point particles, andr_{\rm mat} is the r.m.s. matter radius. The numbers in parentheses are from the DFE calculations using the Nijmegen model D (ND)YY interaction [14].\Delta shows the accuracy of satisfaction of the Pythagorean theorem in Eq. (16).\Delta = \langle r_{(YY)\alpha} \rangle^2 + \frac{1}{4} \langle r_{YY} \rangle^2 - \langle r_{Y\alpha} \rangle^2 = 0.
(16) In Table 6, we present the contribution of different channels, indicated by the quantum numbers
\{ K, l_{x_i}, l_{y_i}, l, S_{x_i}, j_{ab}\} , to the total norm of 3B ground state wave functions of\Lambda\Lambda\alpha and\Omega\Omega\alpha systems in both(Y\alpha-Y) and(YY-\alpha) Jacobi coordinates. As one can see, the main contributions in 3B wave functions come from thes -wave channels, whereas the higher partial wave channels substantially have an insignificant contribution.K 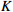
l_{x_i} 
l_{y_i} 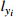
l 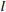
S_{x_i} 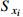
j_{ab} 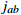
W 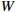
(\Lambda\alpha-\Lambda) Jacobi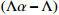
0 0 0 0 0.0 0.0 0.980 2 0 0 0 0.0 0.0 0.004 4 2 2 0 0.0 0.0 0.012 (\Lambda\Lambda-\alpha) Jacobi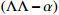
0 0 0 0 0.5 0.5 0.980 2 1 1 0 0.5 0.5 0.004 4 1 1 0 0.5 0.5 0.001 4 0 0 0 0.5 0.5 0.010 (\Omega\alpha-\Omega) Jacobi
0 0 0 0 0.0 0.0 0.993 2 0 0 0 0.0 0.0 0.005 (\Omega\Omega-\alpha) Jacobi
0 0 0 0 1.5 1.5 0.993 2 1 1 0 1.5 1.5 0.005 Table 6. Contributions of different partial wave channels
W to the total norm of 3B ground state wave functions of\Lambda\Lambda\alpha and\Omega\Omega\alpha systems. For each system, the upper panel shows the contributions in(Y\alpha-Y) Jacobi coordinates, and the lower panel shows the contributions in(YY-\alpha) Jacobi coordinates. Channels with a contribution greater than 0.001% are listed.In Fig. 3, we show the first three dominant hyperradial components
\rho^{5/2}R_{\beta}(\rho) for the ground state wave functions of\Lambda\Lambda\alpha and\Omega\Omega\alpha systems, obtained byi_{\max} =25 . As the binding energy of the 3B system increases from the left to right panel, the system becomes more compact in the configuration space. In Fig. 4, we illustrate the probability density of 3B ground states of\Lambda\Lambda\alpha and\Omega\Omega\alpha systems as a function of\langle r_{YY} \rangle , the distance between the two identicalY hyperons, andr_{(YY) \alpha} , the distance between the\alpha particle and the center of mass of twoY particles. As we can see for both systems, the distributions along the\langle r_{YY} \rangle direction are broader than those along ther_{(YY) \alpha} direction,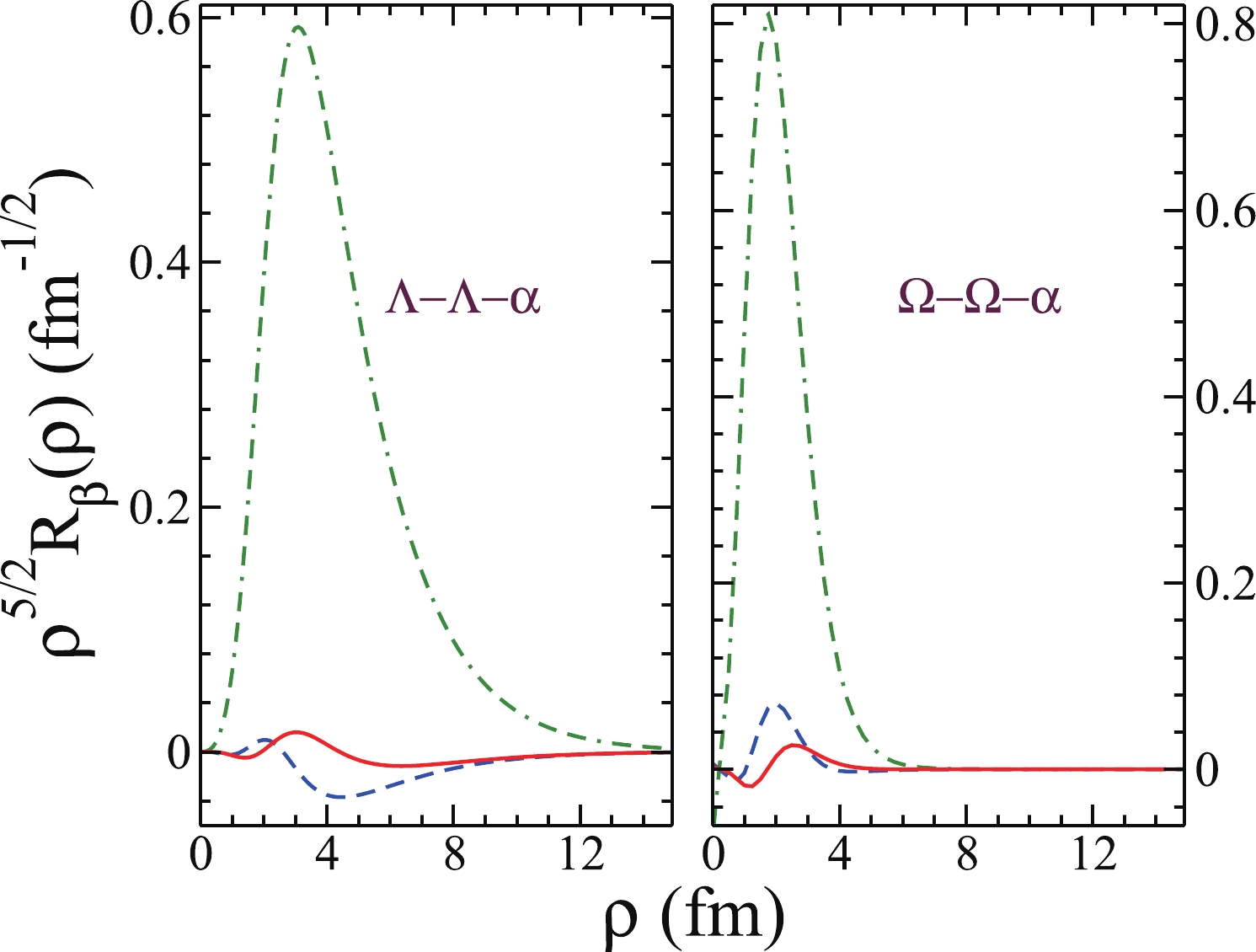
Figure 3. (color online) Hyperradial wave function
\rho^{5/2}R_{\beta}(\rho) for the first three dominant channels,\beta \equiv {0, 0, 0, 0, 0, 0} (green dash-dotted line),\beta \equiv {2, 0, 0, 0, 0, 0} (blue dashed line), and\beta \equiv {4, 0, 0, 0, 0, 0} (red solid line), of the ground state wave functions of\Lambda\Lambda\alpha and\Omega\Omega\alpha systems.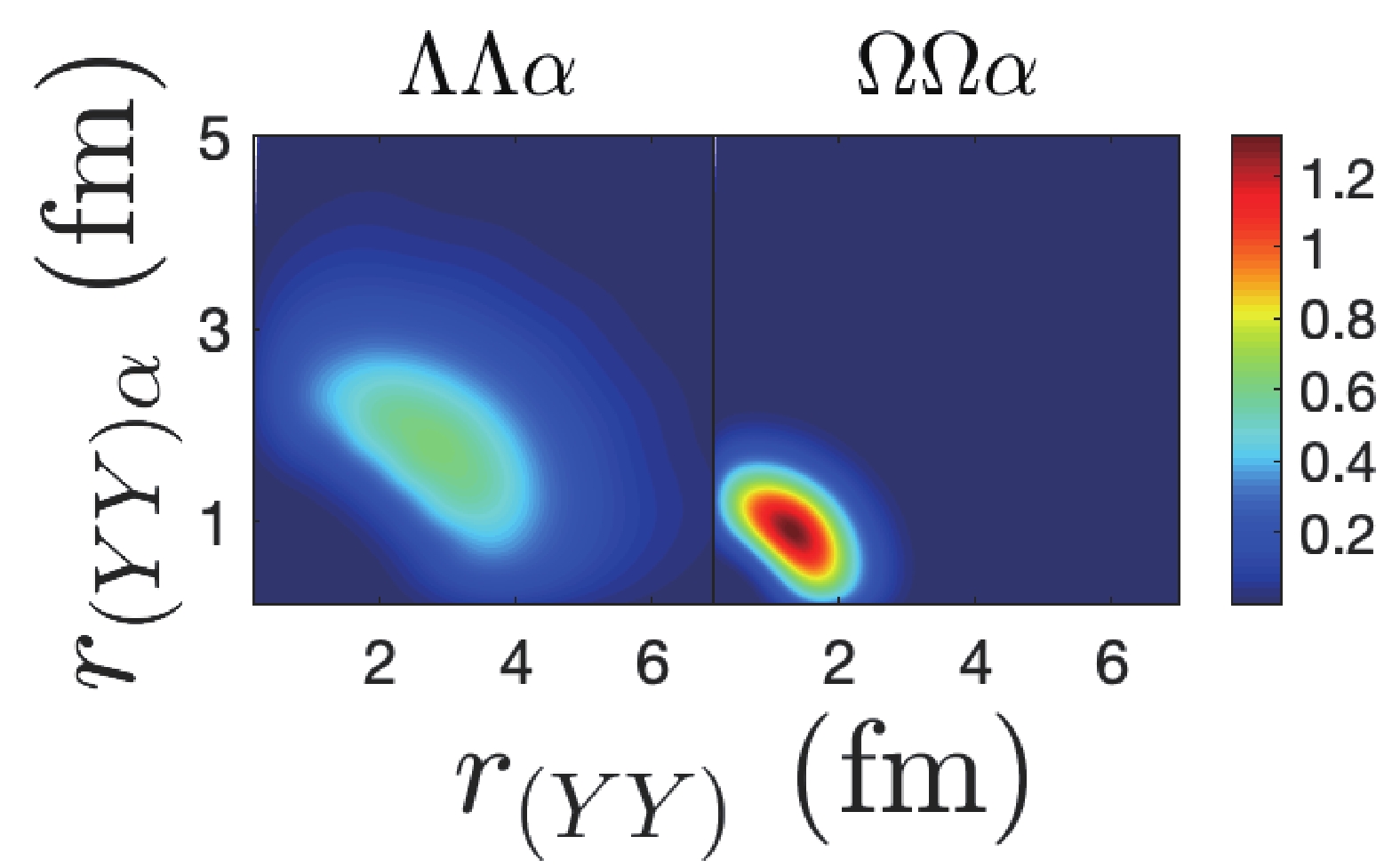
Figure 4. (color online) 3B ground state probability density of
\Lambda\Lambda\alpha and\Omega\Omega\alpha systems as a function of\langle r_{YY} \rangle , the distance betweenYY pair, andr_{(YY) \alpha} , the distance between\alpha particle and the center of mass of theYY pair.confirming that the distance between identical
Y hyperons is greater than the distance between the spectator\alpha particle and the center of mass of theYY pair. -
In this study, we invetigated the ground-state properties of multi-strangeness hypernuclei
{}_{\Lambda\Lambda }^{\;\;\;6}{{\rm{He}}} and{}_{\Omega\Omega }^{\;\;\;6}{{\rm{He}}} in a hyperharmonic three-body model of(YY \alpha) . For this, we solved two coupled Faddeev equations in configuration space with the hyperspherical harmonics expansion method using the most modern two-body interactions, including the recent lattice QCD potentials, to calculate the ground state binding energies and geometrical properties. In our numerical analysis, we checked the convergence of 3B ground state binding energies and nuclear matter radii as a function of the maximum value of hyperradial excitationsi_{\max} and hyperangular quantum numberK_{\max} . Our numerical results show that the ground state binding energy of{}_{\Lambda\Lambda }^{\;\;\;6}{{\rm{He}}} using three models of\Lambda\Lambda lattice interactions changes in the domain of(-7.468 , \ -7.804) MeV, while{}_{\Omega\Omega }^{\;\;\;6}{{\rm{He}}} has a deep binding energy of-67.21 MeV. We should indicate that the implemented\Omega \alpha potential in our calculations is restricted to one angular momentum channel only, whereas the contribution of repulsive channels is not considered. Thus, this could explain the deep binding of the\Omega \alpha and consequently the{}_{\Omega\Omega }^{\;\;\;6}{{\rm{He}}} system.We studied the geometrical properties of the aforementioned
^6 He double hyperon by calculating the expectation values of the Jacobi coordinates and the r.m.s. matter radius and correlation density. Our numerical results confirm that the studied 3B systems, composed of two identical hyperons and one alpha particle, form isosceles triangles, where the most probable positions of the particles perfectly satisfy the Pythagorean theorem. Our numerical analysis on the structure of 3B ground state wave functions shows that the main contributions of over 99% are from thes -wave channels. Our numerical results for{}_{\Lambda\Lambda }^{\;\;\;6}{{\rm{He}}} are in agreement with the results of other theoretical studies.Considering the contributions of the coupled channels in
\Lambda \Lambda- \Xi N (\Omega N-\Lambda\Xi - \Sigma \Xi ) interactions is a complementary task to be implemented in the FaCE toolkit to include the coupled components in the wave function of{}_{\Lambda\Lambda }^{\;\;\;6}{{\rm{He}}} ({}_{\Omega\Omega }^{\;\;\;6}{{\rm{He}}} ). As shown in Refs. [81, 84], the contribution of the coupled channels leads to an increase of approximately0.1 \sim 0.4 MeV in the\Lambda\Lambda\alpha binding energy, while using an effective single-channel interaction leads to a reduction of approximately0.3 MeV [14]. This reduction is due to the tight\alpha cluster binding, which inhibits the effectiveness of the\Lambda \Lambda-\Xi N coupling. We assume that this should also be valid for the\Omega \Omega \alpha system. Moreover, while the contribution of transition potentials to the inelastic channels [85], i.e.,\Omega N-\Lambda\Xi - \Sigma \Xi , are expected to be small [79], they have not yet been derived from the lattice QCD calculations and can be considered in a future study when they are developed. -
F.E. thanks T. Hatsuda and J. Casal for helpful discussions and suggestions.







 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF
















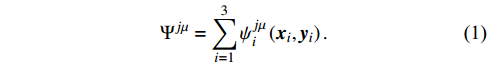

 DownLoad:
DownLoad:
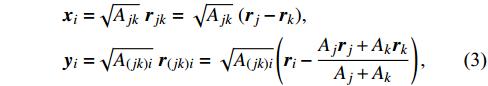
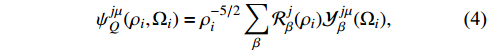

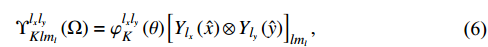
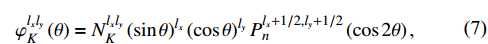
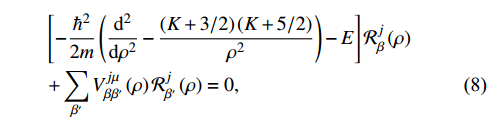
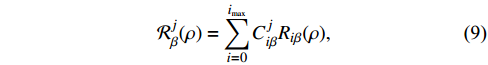
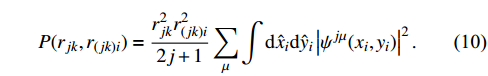
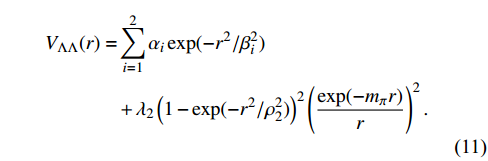
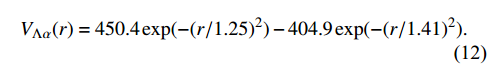
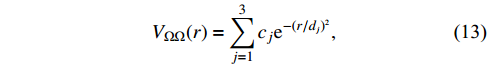
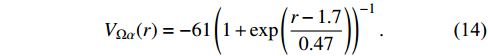
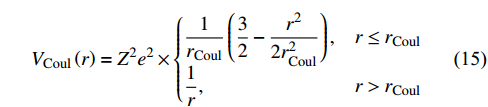
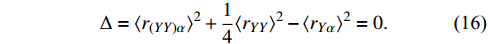











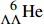
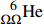
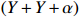
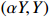
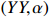
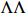
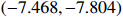
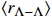
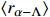
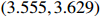
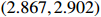

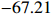
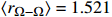





 DownLoad:
DownLoad: